GRAPHING LINES USING INTERCEPTS

Find the x and y intercept of the line with the given equation.

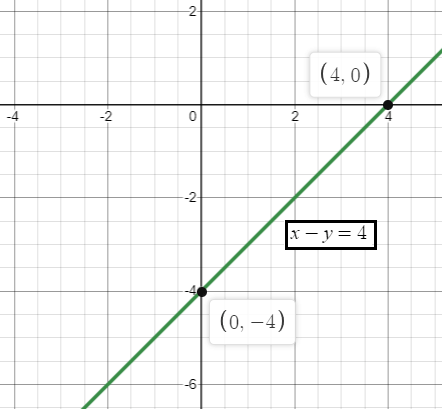
Problem 1 :

x - y = 4

Solution : 

To find x and y intercept we have to compare given equation with intercept form

(x/a) + (y/b) = 1

x - y = 4

Dividing by 4 on both sides,

x/4 - y/4 = 4/4

(x/4) - (y/4) = 1

x -intercept (a) = 4

y -intercept (b) = - 4

The required points are (4, 0) and (0, -4).

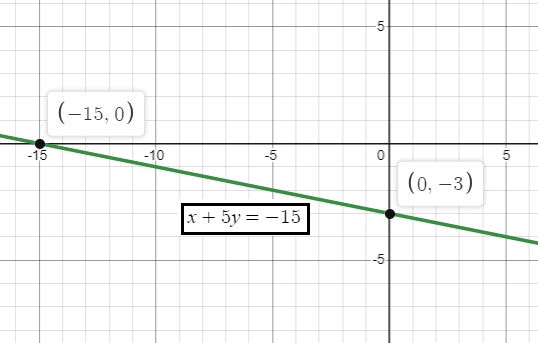
Problem 2 :

x + 5y = -15

Solution :

To find x and y intercept we have to compare given equation with intercept form

(x/a) + (y/b) = 1

x + 5y = -15

Dividing by -15 on both sides,

(x/-15) + (5y/-15) = -15/-15

(x/-15) + (y/-3) = 1

x -intercept (a) = - 15

y -intercept (b) = - 3

So, the points are (-15, 0) and (0, -3).

Problem 3 :

3x - 4y = -12

Solution :

To find x and y intercept we have to compare given equation with intercept form

(x/a) + (y/b) = 1

3x - 4y = -12

Dividing by -12 on both sides,

(3x/-12) - (4y/-12) = -12/-12

(x/-4) - (y/-3) = 1

x -intercept (a) = - 4

y -intercept (b) = 3

So, the points are (-4, 0) and (0, 3).

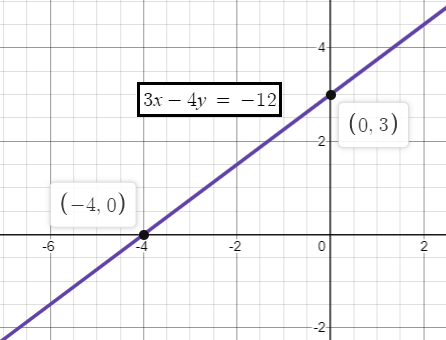
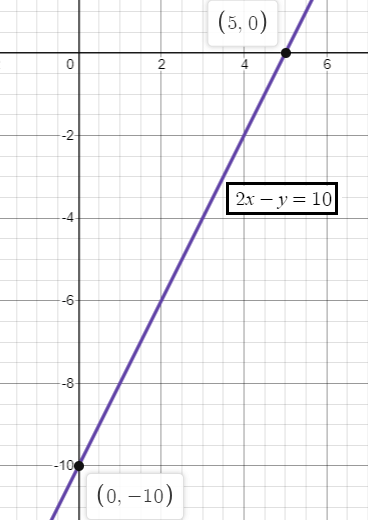
Problem 4 :

2x - y = 10

Solution :

To find x and y intercept we have to compare given equation with intercept form

(x/a) + (y/b) = 1

2x - y = 10

Dividing by 10 on both sides,

(2x/10) - (y/10) = 10/10

(x/5) - (y/10) = 1

x -intercept (a) = 5

y -intercept (b) = - 10

So, the points are (5, 0) and (0, -10).

Problem 5 :

4x - 5y = 20

Solution :

To find x and y intercept we have to compare given equation with intercept form

(x/a) + (y/b) = 1

4x - 5y = 20

Dividing by 20 on both sides,

(4x/20) - (5y/20) = 20/20

(x/5) - (y/4) = 1

x -intercept (a) = 5

y -intercept (b) = -4

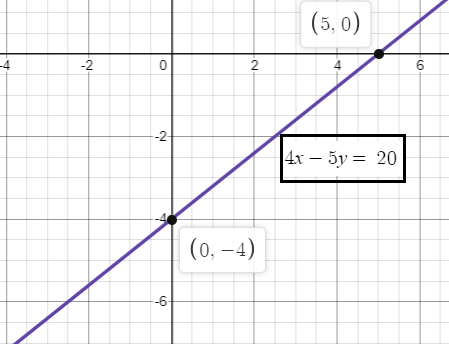
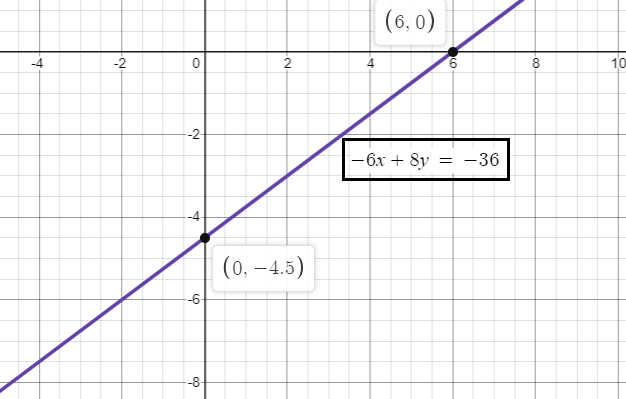
Problem 6 :

-6x + 8y = -36

Solution :

To find x and y intercept we have to compare given equation with intercept form

(x/a) + (y/b) = 1

-6x + 8y = -36

Dividing by -36 on both sides,

(-6x/-36) + (8y/-36) = -36/-36

(x/6) + (2y/-9) = 1

x -intercept (a) = 6

y -intercept (b) = - 9/2