GRAPHING LINEAR INEQUALITIES

To obtain the common region for both given linear inequalities, we have to follow the steps given below.

Step 1 :

First, we have to assume the given inequalities as equations.

Then, we have to draw the graph of the line using x and y-intercepts.

Step 2 :

The region which satisfies the inequality can be shaded.

To check if the inequality is being satisfied by applying any point from the region, the point which satisfies the inequality is known as the solution region and that can be shaded.

Step 3 :

Do the process explained in step 2 for the second inequality.

Step 4 :

The overlapping region is the solution region for the system of inequalities given.

Example 1 :

Graph the system of linear inequalities.

y ≥ 1/2x + 3

y ≥ x - 2

Solution :

Given,

y ≥ 1/2x + 3 and y ≥ x - 2

Converting the inequalities into equations.

y = 1/2x + 3 and y = x - 2

To graph the line y = 1/2x + 3, we find x and y intercepts.

x-intercept :

Put y = 0

y = 1/2x + 3

0 = 1/2x + 3

-1/2x = 3

x = -6

y-intercept :

Put x = 0

y = 1/2x + 3

y = 1/2(0) + 3

y = 3

To graph the line y = x - 2, we find x and y intercepts.

x-intercept :

Put y = 0

y = x - 2

0 = x - 2

x = 2

y-intercept :

Put x = 0

y = x - 2

y = 0 - 2

y = -2

The point on the line y = 1/2x + 3 are (-6, 0) and (0, 3)

The point on the line y = x - 2 are (2, 0) and (0, -2)

By plotting the points on the graph, we get

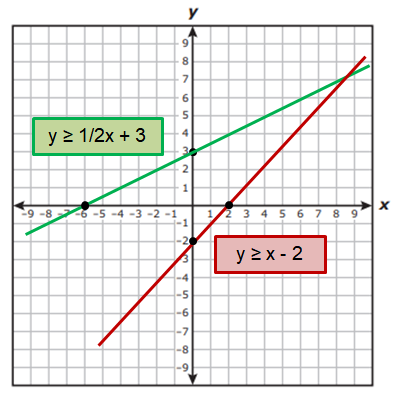
If x = 1 and y = 4, then y ≥ 1/2x + 3 (True)

So, we can shade the region which is above to y ≥ 1/2x + 3.

If x = -7 and y = 4, then y ≥ x - 2 (True)

So, we can shade the region which is above to y ≥ x - 2.

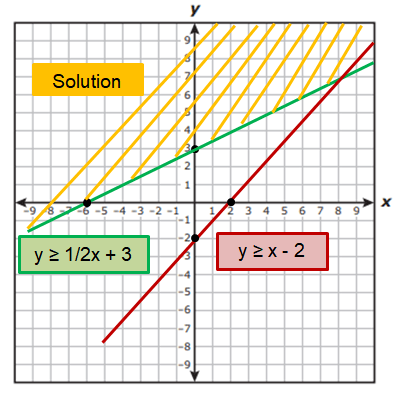
Example 2 :

Graph the system of linear inequalities.

y ≥ 2x + 3

y ≤ x - 2

Solution :

Given,

y ≥ 2x + 3 and y ≤ x - 2

Converting the inequalities into equations.

y = 2x + 3 and y = x - 2

To graph the line y = 2x + 3, we find x and y intercepts.

x-intercept :

Put y = 0

y = 2x + 3

0 = 2x + 3

-2x = 3

x = -3/2

y-intercept :

Put x = 0

y = 2x + 3

y = 0 + 3

y = 3

To graph the line y = x - 2, we find x and y intercepts.

x-intercept :

Put y = 0

y = x - 2

0 = x - 2

x = 2

y-intercept :

Put x = 0

y = x - 2

y = 0 - 2

y = -2

The point on the line y = 2x + 3 are (-3/2, 0) and (0, 3)

The point on the line y = x - 2 are (2, 0) and (0, -2)

By plotting the points on the graph, we get

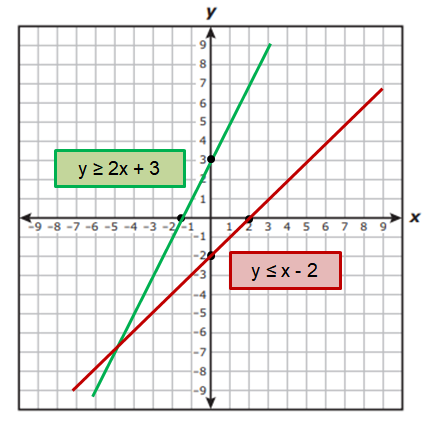
If x = -3 and y = 4, then y ≥ 2x + 3 (True)

So, we can shade the region which is left to y ≥ 2x + 3.

If x = 4 and y = -4, then y ≤ x - 2 (True)

So, we can shade the region which is right to y ≤ x - 2.

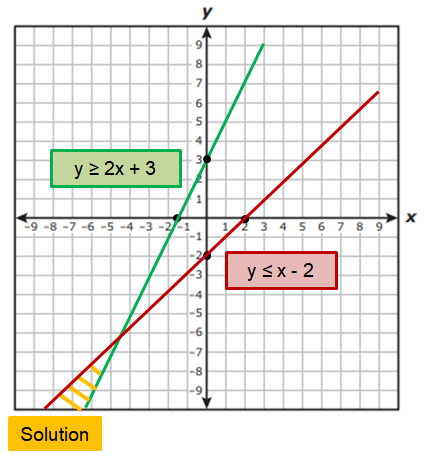
Example 3 :

Write the inequality for the graph given below.

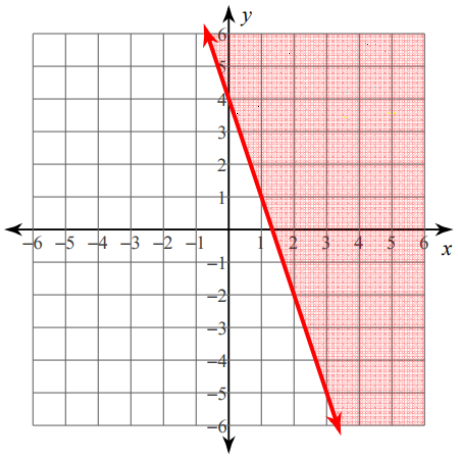
Solution :

From the above graph, first let us find the slope and y-intercept.

Rise = - 3 and Run = 1

Slope = - 3 / 1 = - 3

y-intercept = 4

So, the equation of the given line is

y = - 3x + 4

But, we need to use inequality which satisfies the shaded region.

Because the graph contains solid line, we have to use one of the signs ≤ or ≥.

To find the correct sign, let us take a point from the shaded region.

Take the point (2, 1) and substitute into the equation of the line.

y = - 3x + 4

That is,

1 = - 3(2) + 4

1 = - 6 + 4

1 = - 2

Here, 1 is greater than -2. So, we have to choose the sign ≥ instead of equal sign in the equation y = -3x + 4

Therefore, the required inequality is

y ≥ - 3x + 4.

GRAPHING LINEAR INEQUALITIES

Recent Articles

Finding Range of Values Inequality Problems

Solving Two Step Inequality Word Problems

Exponential Function Context and Data Modeling