FROM THE GIVEN INFORMATION FIND EQUATION OF ELLIPSE

Find the standard form of the equation of each ellipse.

Problem 1 :

Foci (0, ±3), vertices (0, ±5)

Solution:

Given, 

Vertices: (0, ±5)

The vertices are of the form (0, ±a)

a = 5

Hence, the major axis along y-axis.

Foci = (0, ±c)

= (0, ±3)

c = 3

b² = a² - c²

b² = 5² - 3²

b² = 25 - 9

b² = 16

Thus, the equation of the ellipse is

Problem 2 :

Major axis horizontal with length 12,length of minor axis 4; center: (-1, 3)

Solution:

Major axis is horizontal

Center (h, k) = (-1, 3)

Major axis 2a = 12

a = 6

Minor axis 2b = 4

b = 2

Thus, the equation of the ellipse is

Problem 3 :

Foci (±5, 0), length of major axis 12

Solution:

Given, Foci (±5, 0)

length of major axis = 12

The foci are on the x-axis the major axis is along the x-axis.

Major axis 2a = 12

a = 6

Foci = (±c, 0)

c = 5

b² = a² - c²

= 6² - 5²

= 36 - 25

b² = 11

Thus, the equation of the ellipse is

Problem 4 :

Endpoints of major axis: (2, 2) & (8, 2), Endpoints of minor axis: (5, 3) & (5, 1)

Solution:

This is an ellipse with horizontal major axis of the standard form:

Length of major axis = 8 - 2 = 6

2a = 6

a = 3

Length of minor axis = 3 - 1 = 2

2a = 2

a = 1

Thus, the equation of the ellipse is

Problem 5 :

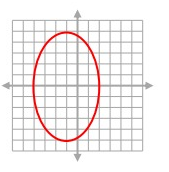
Solution:

Major axis = (-1, 5) (-1, -5)

Minor axis = (2, 0) (-4, 0)

This is an ellipse with vertical major axis of the standard form:

Length of major axis a = 5 

Length of minor axis b = 3

Thus, the equation of the ellipse is

Problem 6 :

Solution:

Major axis = (5, -1) (-1, -1)

Minor axis = (2, 1) (2, -3)

This is an ellipse with horizontal major axis of the standard form:

Length of major axis a = 3

Length of minor axis b = 2

Thus, the equation of the ellipse is