FINDING X INTERCEPTS OF A QUADRATIC FUNCTION

The graphical form of any quadratic function will be a parabola (the shape of U).

The quadratic equations which is in the form of

y = ax² + bx + c

may be open upward parabola or open downward parabola.

Based on the sign of leading coefficient, we may decide the parabola opens up or down.

How to find x-intercept ?

By applying y = 0, we can find the x-intercept.

If the quadratic equation is having two distinct real roots, the parabola will pass through those different points on the x-axis.

Graph crosses x-axis twice

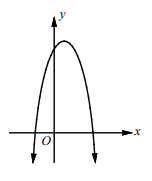
If the quadratic equation is having two same real roots, the parabola will intersect the x-axis once. (Multiplicity)

Graph crosses x-axis once

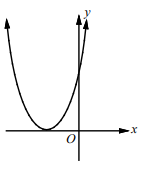
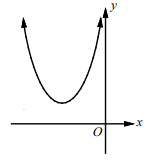
If the quadratic equation is having no real roots, the parabola will not intersect the x-axis.

Graph does not cross x-axis

To solve quadratic equation, we have three different ways.

i) Using factoring

ii)  Using quadratic formula

iii) Using completing the square.

Find the x-intercepts for:

Problem 1 :

y = x² - 9

Solution :

y = x² - 9

To find x-intercept put y = 0

x² - 9 = 0

x² = 9

x = ±3

So, x-intercepts are 3 and -3.

Problem 2 :

y = 2x² - 6

Solution :

y = 2x² - 6

To find x-intercept put y = 0

2x² - 6 = 0

2x² = 6

x² = 3

x = ±√3

So, x-intercepts are √3 and -√3.

Problem 3 :

y = x² + 7x + 10

Solution :

y = x² + 7x + 10

To find x-intercept put y = 0

x² + 7x + 10 = 0

(x + 5) (x + 2) = 0

x + 5 = 0     x + 2 = 0

x = -5 and x = -2

So, x-intercepts are -5 and -2.

Problem 4 : 

y = x² + x - 12

Solution :

y = x² + x - 12

To find x-intercept put y = 0

x² + x - 12 = 0

x² - 3x + 4x - 12 = 0

(x - 3) (x + 4) = 0

x - 3 = 0    x + 4 = 0

x = 3 and x = -4

So, x-intercepts are 3 and -4.

Problem 5 :

y = 4x - x²

Solution :

y = 4x - x²

To find x-intercept put y = 0

4x - x² = 0

x(4 - x) = 0

x = 0   4 - x = 0

 -x = -4

x = 4

So, x-intercepts are 0 and 4.

Problem 6 :

y = -x² - 6x - 8

Solution :

y = -x² - 6x - 8

To find x-intercept put y = 0

-x² - 6x - 8 = 0

-(x² + 6x + 8) = 0

-(x² + 2x + 4x + 8) = 0

(x + 4) (x + 2) = 0

x + 4 = 0    x + 2 = 0

x = -4          x = -2

So, x-intercepts are -4 and -2.

Problem 7 :

y = -2x² - 4x - 2

Solution :

y = -2x² - 4x - 8

To find x-intercept put y = 0

-2x² - 4x - 8 = 0

Using the quadratic formula,

x = -b ± √b² - 4ac

a = -2, b = -4 and c = -8

x = 4 ± √(-4)² - 4(-2)(-2) / 2(-2)

= 4 ± √16 -16 / -4

= 4 ± √0 / -4

= 4 / -4

x = -1

So, x-intercept is -1.

Problem 8 :

y = 4x² - 24x + 36

Solution :

y = 4x² - 24x + 36

To find x-intercept put y = 0

4x² - 24x + 36 = 0

Using the quadratic formula,

x = -b ± √b² - 4ac

a = 4, b = -24 and c = 36

x = 24 ± √(-24)² - 4(4)(36) / 2(4)

= 24 ± √576 -576 / 8

= 24 ± √0 / 8

= 24 / 8

x = 3

So, x-intercept is 3.

Problem 9 :

y = x² - 4x + 1

Solution :

y = x² - 4x + 1

To find x-intercept put y = 0

x² - 4x + 1 = 0

Using the quadratic formula,

x = -b ± √b² - 4ac

a = 1, b = -4 and c = 1

x = 4 ± √(-4)² - 4(1)(1) / 2(1)

= 4 ± √16 - 4 / 2

= 4 ± √12 / 2

= 4 ± 2√3 / 2

x = 2 ± √3

So, x-intercepts are 2 ± √3.

Problem 10 :

y = x² + 4x - 3

Solution :

y = x² + 4x - 3

To find x-intercept put y = 0

x² + 4x - 3 = 0

Using the quadratic formula,

x = -b ± √b² - 4ac

a = 1, b = 4 and c = -3

x = -4 ± √(4)² - 4(1)(-3) / 2(1)

= -4 ± √16 + 12 / 2

= -4 ± √28 / 2

= -4 ± 2√7 / 2

x = -2 ± √7

So, x-intercepts are -2 ± √7.

Problem 11 :

y = x² - 6x - 2

Solution :

y = x² - 6x - 2

To find x-intercept put y = 0

x² - 6x - 2 = 0

Using the quadratic formula,

x = -b ± √b² - 4ac

a = 1, b = -6 and c = -2

x = 6 ± √(-6)² - 4(1)(-2) / 2(1)

= 6 ± √36 + 8 / 2

= 6 ± √44 / 2

= 6 ± 2√11 / 2

x = 3 ± √11

So, x-intercepts are 3 ± √11.

Problem 12 :

y = x² + 8x + 11

Solution :

y = x² + 8x + 11

To find x-intercept put y = 0

x² + 8x + 11 = 0

Using the quadratic formula,

x = -b ± √b² - 4ac

a = 1, b = 8 and c = 11

x = -8 ± √(8)² - 4(1)(11) / 2(1)

= -8 ± √64 - 44 / 2

= -8 ± √20 / 2

= -8 ± 2√5 / 2

x = -4 ± √5

So, x-intercepts are -4 ± √5.