FINDING THE TURNING POINT OF A QUADRATIC FUNCTION

What is vertex ?

The graph of the quadratic function is called a parabola. The point where the graph turns is called the vertex.

If the graph opens upward, the y-coordinate of the vertex is the minimum and the graph is concave upwards.

If the graph opens downward, the y-coordinate of the vertex is the maximum and the graph is concave downwards.

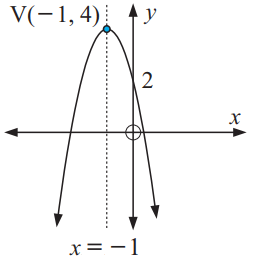
To find vertex of a parabola, we have two different ways.

1) Using completing the square

2) Using formula

Find the turning point of the quadratic functions given below.

Problem 1 :

y = x² + 7x + 10

Solution :

y = x² + 7x + 10

Using completing the square method :

Turning point is at (7/2, -9/4).

Using formula :

y = x² + 7x + 10

Here a = 1, b = 7 and c = 10

Turning point is at (7/2, -9/4).

Problem 2 :

y = x² + x - 12

Solution :

y = x² + x - 12

Using completing the square method :

The turning point is (1/2, -49/4).

Using formula :

x-coordinate of turning point is x = -b/2a

Here a = 1, b = 1 and c = -12.

The turning point is (1/2, -49/4).

Problem 3 :

y = 4x - x²

Solution :

y = - x² + 4x

Using completing the square method :

y = - [x² - 4x]

y = - [x² - 2⋅ x ⋅ 2 + 2² - 2²]

y = - [(x - 2)² - 2²]

y = - [(x - 2)² - 4]

y = - (x - 2)² + 4

(h, k) ==> (2, 4)

Using formula :

So, the turning point is at (2, 4).

Problem 4 :

y = -2x² - 4x - 2

Solution :

Using completing the square method :

y = -2[x² + 2x + 1]

y = -2 [x² + 2⋅ x ⋅ 1 + 1² - 1² + 1]

y = - [(x + 1)² -1 + 1]

y = - [(x + 1)² + 0]

(h, k) ==> (-1, 0)

Using formula :

x = -b/2a

Here a = -2, b = -4 and c = -2.

So, the turning point is at (-1, 0).

Problem 5 :

y = 4x² - 24x + 36

Solution :

Using completing the square method :

y = 4[x² - 6x + 9]

y = 4 [x² - 2⋅ x ⋅ 3 + 3² - 3² + 9]

y = 4[(x - 3)² -9 + 9]

y = - 4[(x - 3)² + 0]

y = - 4(x - 3)² + 0

(h, k) ==> (3, 0)

Using formula :

Here a = 4, b = -24 and c = 36

So, the turning point is (3, 0).

Problem 6 :

y = x² - 4x + 1

Solution :

Using completing the square method :

y = [x² - 4x + 1]

y = [x² - 2⋅ x ⋅ 2 + 2² - 2² + 1]

y = [(x - 2)² -4 + 1]

y = [(x - 2)² - 3]

(h, k) ==> (2, -3)

Using formula :

Here a = 1, b = -4 and c = 1.

x = 4/2(1)

x = 4/2

x = 2

y- Intercept: (x = 2)

y = (2)² - 4(2) + 1

y = 4 - 8 + 1

y = -3

So, the turning point is at (2, -3).

Problem 7 :

y = x² + 4x - 3

Solution :

Using completing the square method :

y = [x² + 4x - 3]

y = [x² - 2⋅ x ⋅ 2 + 2² - 2² - 3]

y = [(x - 2)² -4 - 3]

y = [(x - 2)² - 7]

(h, k) ==> (2, -7)

Using formula :

Here a = 1, b = 4 and c = -3.

So, the turning point is at (-2, -7).