FINDING THE MISSING DIAGONAL OF A RHOMBUS

Find the length of the missing diagonal in each rhombus.

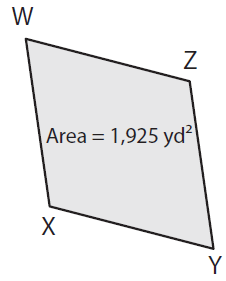
Problem 1 :

If WY = 70 yd, find XZ.

Solution :

Given, Area = 1925 yd² and WY = 70 yd

Area of the rhombus = 1/2 × d₁ × d₂

Here d₁ = WY and  d₂ = XZ

Area = 1/2 × WY × XZ 

1925 yd² = 1/2 × 70 yd × d₂

1925 yd² = 35 yd × d₂ 

(1925 yd²) / 35 yd = d₂ 

XZ = d₂ = 55 yd

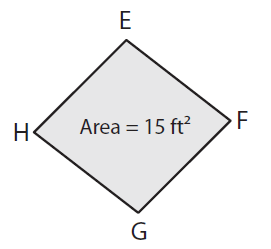
Problem 2 :

If FH = 6 ft, find EG.

Solution :

Given, Area = 15 ft² and FH = 6 ft

Area of the rhombus = 1/2 × d₁ × d₂

Here FH = d₁ and EG = d₂

Area = 1/2 × FH × EG

15 ft² = 1/2 × 6 ft × d₂ 

15 ft² = 3 ft × d₂ 

(15 ft²) / 3 ft = d₂ 

EG = d₂ = 5 ft

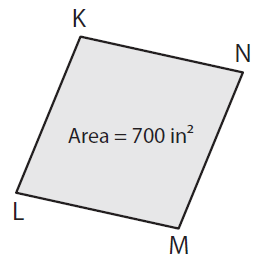
Problem 3 :

If KM = 35 in, find LN.

Solution :

Given, Area = 700 in² and KM = 35 in

Area of the rhombus = 1/2 × d₁ × d₂

Here d₁ = KM and  d₂ = LN

Area = 1/2 × KM × LN

700 in² = 1/2 × 35 in × d₂

 (700 in² × 2) / 35 in = d₂

(1400 in²) / 35 in = d₂

LN = d₂ = 40 in

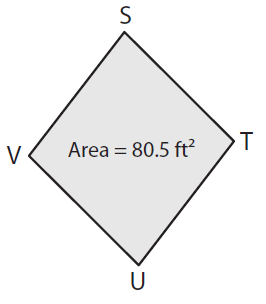
Problem 4 :

If VT = 7ft, find SU.

Solution :

Given, Area = 80.5 ft² and VT = 7 ft

Area of the rhombus = 1/2 × d₁ × d₂

Here d₁ = VT and  d₂ = SU

Area = 1/2 × VT × SU

80.5 ft² = 1/2 × 7 ft × d₂

(80. 5 ft² × 2) / 7 ft =  d₂

d₂ = (161 ft²) / 7 ft

SU = d₂ = 23 ft

Problem 5 :

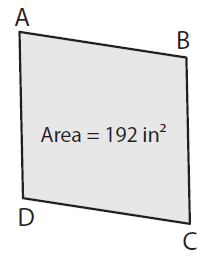
If BD = 16 in, find AC.

Solution :

Given, Area = 192 in² and BD = 16 in

Area of the rhombus = 1/2 × d₁ × d₂

Here d₁ = BD and  d₂ = AC

Area = 1/2 × BD × AC

192 in² = 1/2 × 16 in × d₂

192 in² =  8 in × d₂

d₂ = (192 in²) / 8 in

AC = d₂ = 24 in

Problem 6 :

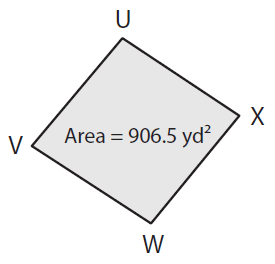
If VX = 49 yd, find UW.

Solution :

Given, Area = 906.5 yd² and VX = 49 yd

Area of the rhombus = 1/2 × d₁ × d₂

Here d₁ = VX and  d₂ = UW

Area = 1/2 × VX × UW

906.5 yd² = 1/2 × 49 yd × d₂

(906.5 yd² × 2) / 49 yd = d₂ 

(1813 yd²) / 49 yd = d₂

UW = d₂ = 37 yd

Problem 7 :

The length of one of the diagonal of a rhombus is 38 inches. Find the length of the other diagonal, if the area is 646 square inches.

Solution :

Given, Area = 646 square inches

Length of one diagonal d₁ = 38 inches

Length of other diagonal d₂ = ?

Area of the rhombus = 1/2 × d₁ × d₂

646 in² = 1/2 × 38 in × d₂

646 in² = 19 in × d₂

(646 in²) / 19 in = d₂

d₂ = 34 inches

So, the length of other diagonal of a rhombus is 34 in.

Problem 8 :

The area of a rhombus is 125 square yards. If one of the diagonals measures 10 yards, find the length of the other diagonal.

Solution :

Given, Area = 125 square yards

Length of one diagonal d₁ = 10 yards

Length of other diagonal d₂ = ?

Area of the rhombus = 1/2 × d₁ × d₂

125 yd² = 1/2 × 10 yd × d₂

125 yd² = 5 yd × d₂

(125 yd²) / 5 yd = d₂

d₂ = 25 yd

So, the length of other diagonal of a rhombus is 25 yd.

Problem 9 :

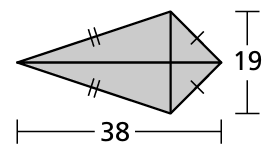
Find the area of the kite or rhombus.

Solution :

Length of diagonals are 38 and 19

Area of kite or rhombus = (1/2) x d1 x d2

= (1/2) x 38 x 19

= 19 x 19

= 361 square units.

Problem 10 :

Find the area of the kite or rhombus.

Solution :

Length of diagonal 1 = 7 + 7 ==> 14 inches

Length of diagonal 2 = 10 + 10 ==> 20 inches

Area of rhombus = (1/2) x 14 x 20

= 7 x 20

= 140 square inches

Problem 11 :

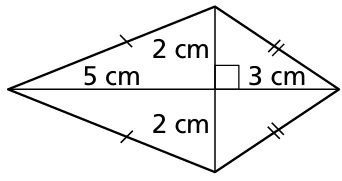
Find the area of the kite or rhombus.

Solution :

Length of diagonal 1 = 5 + 3 ==> 8 cm

Length of diagonal 2 = 2 + 2 ==> 4 cm

Area of rhombus = (1/2) x 8 x 4

= 4 x 4

= 16 cm²

Problem 12 :

Find the area of the kite or rhombus.

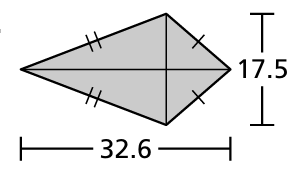
Solution :

Length of diagonal 1 = 32.6 inches

Length of diagonal 2 = 17.5 inches

Area of kite = (1/2) x 32.6 x 17.5

= 16.3 x 17.5

= 285.25 square inches.

Problem 13 :

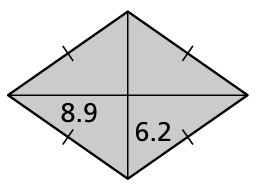
Solution :

Length of diagonal 1 = 8.9 + 8.9

= 17.8

Length of diagonal 2 = 6.2 + 6.2

= 12.4

Area of kite = (1/2) x 12.4 x 17.8

= 6.2 x 17.8

= 110.36 square inches.

Problem 14 :

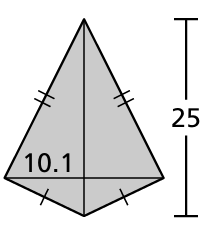
Solution :

Length of diagonal 1 = 25

Length of diagonal 2 = 10.1 + 10.1

= 20.2

Area of kite = (1/2) x 20.2 x 25

= 10.1 x 25

= 252.5 square inches

= 110.36 square inches.

Problem 15 :

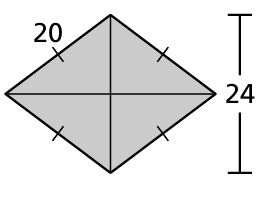
Solution :

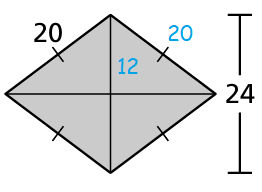
In the triangle above, the base of the triangle be x.

20² = 12² + x²

400 = 144 + x²

x² = 400 - 144

x² = 256

x = 16

Length of diagonal 1 = 24

length of diagonal 2 = 16 + 16 ==> 32

Area of rhombus = (1/2) x 24 x 32

= 12 x 32

= 384 square units.