FINDING THE DOMAIN OF A RATIONAL FUNCTION WITH SQUARE ROOT
Domain is set of possible inputs.
Case 1 :
When we have square root function at the numerator, the value that we receive after applying possible inputs should never become negative.
Example :
Solution :
Case 2 :
When we have square root function at the denominator, the value that we receive after applying possible inputs should never become negative as well 0.
Example :
Solution :
So, domain is (-∞,-2) and (2, ∞).
Case 3 :
When we have square root function in both numerator and denominator, the value that we receive after applying possible inputs should never become negatives and the denominator should not become 0.
Example :
Solution :
So, domain is [2, ∞).
Find the domain of the functions given below.
Problem 1 :
Solution:
Equating the denominator to 0, we get
√(x + 2) - 3 = 0
√(x + 2) = 3
x + 2 = 32
x + 2 = 9
x = 7
x ≠ 7
x + 2 ≥ 0
x ≥ -2
Domain:
{x| x ∈ R, x ≥ -2, x ≠ 7}
Problem 2 :
Solution:
√(9 - x2) = 0
9 - x2 > 0
x2 < 9
x < ± 3
Decomposing into intervals, (-∞, -3)(-3, 3) and (3, ∞).
|
x = -4 ∈ (-∞, -3) 9 - (-4)2 > 0 False |
x = 0 ∈ (-3, 3) 9 - (0)2 > 0 True |
x = 4 ∈ (3, ∞) 9 - 42 > 0 False |
Domain:
(-3, 3)
Problem 3 :
Solution:
√(1 - x) ≥ 0
1 - x ≥ 0
-x ≥ -1
x ≤ 1
√(x - 5) > 0
x > 5
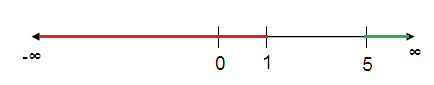
Decomposing into intervals, (-∞, 1)(1, 5) and (5, ∞).
|
x = 0 ∈ (-∞, 1) √(1 - x) ≥ 0 and √(x - 5) > 0 √(1 - 0) ≥ 0 and √(0 - 5) > 0 False |
x = 2 ∈ (1, 5) √(1 - x) ≥ 0 and √(x - 5) > 0 √(1 - 2) ≥ 0 and √(2 - 5) > 0 False |
x = 2 ∈ (5, ∞)
√(1 - x) ≥ 0 and √(x - 5) > 0
√(1 - 2) ≥ 0 and √(2 - 5) > 0
False
Domain:
Ø
Problem 4 :
Solution:
x ≠ 0
√(9 - x2) ≥ 0
9 - x2 ≥ 0
x2 ≤ 9
x ≤ ± 3
Decomposing into intervals, (-∞, -3)(-3, 0), (0, 3) and (3, ∞).
|
x = -4 ∈ (-∞, -3) √(9 - x2) ≥ 0 and x ≠ 0 √(9 - 16) ≥ 0 and x ≠ 0 False |
x = -2 ∈ (-3, 0) √(9 - x2) ≥ 0 and x ≠ 0 √(9 - 4) ≥ 0 and x ≠ 0 True |
|
x = 1 ∈ (0, 3) √(9 - x2) ≥ 0 and x ≠ 0 √(9 - 1) ≥ 0 and x ≠ 0 True |
x = 4 ∈ (3, ∞). √(9 - x2) ≥ 0 and x ≠ 0 √(9 - 16) ≥ 0 and x ≠ 0 False |
Domain:
[-3, 3]
Problem 5 :
Solution:
Equating the denominator to 0, we get
√(x2 - 4) - 3 = 0
√(x2 - 4) = 3
x2 - 4 = 9
x2 = 13
x = √13
x ≠ √13
x2 - 4 ≥ 0
x2 ≥ 4
x ≥ ± 2
Domain:
{x| x ∈ R, x ≥ 2 but x ≠ √13}
Problem 6 :
Solution:
√(x - 8) = 0
x - 8 = 0
x = 8
x ≠ 8
x - 8 > 0
x > 8
Domain:
{x| x ∈ R, x > 8 but x ≠ 8}
Problem 7 :
Solution:
√(x + 3) > 0
x + 3 > 0
x > -3
Domain:
{x| x ∈ R, x > -3}
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling