FINDING MISSING MEASURES OF SIMILAR FIGURES

Problem 1 :

Lance and Alien is 5 feet tall. His shadow is 8 feet long.

At the same time of day, a tree's shadow is 32 feet long. What is the height of the tree ?

Solution :

Let h be the height of the tree.

5/8 = h/32

5(32) = 8h

h = (32/8) x 5

h = 4 x 5

h = 20 feet

Problem 2 :

Pentagon JKLMN is similar to pentagon VWXYZ, what is the measurement of angle X ?

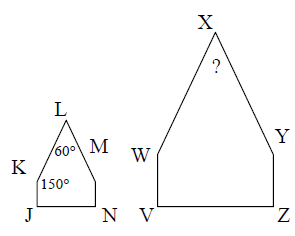
Solution :

Since two shapes are similar, they must be equiangular.

So, WXY = 60 degree.

Problem 3 :

Triangle PQR is similar to triangle DEF as shown.

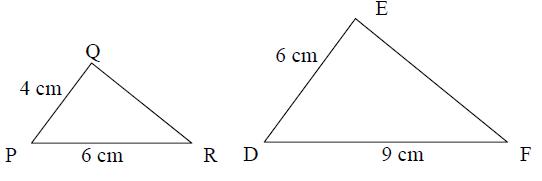
which describes the relationship between the corresponding sides of the two triangles ?

a)  PQ/DE = 4/6        b)  PQ/DE = 6/4

c)  PQ/EF = 4/9        d)  PR/DE = 6/6

Solution :

In the triangles above PQR and EDF, PQ and DE are corresponding sides.

PQ = 4 cm and DE = 6 cm

PQ/DE = 4/6

Problem 4 :

A six foot tall person is standing next to flag pole. The person is casting a shadow 1 1/2 feet in length while a flagpole is casting a shadow 5 feet in length. How tall is the flagpole ?

a)  30 ft     b) 25 ft    c)  20 ft     d)  15 ft

Solution :

Ratio between height of the person and his shadow

6 : 1  1/2 ==> 6 : 3/2

Ratio between height of pole and its shadow.

Let h be the height of the flag pole.

h : 5

then,

 6 : 3/2 = h : 5

6 x (2/3) = h/5

4 = h/5

h = 4(5)

h = 20

So, height of flag pole is 20 feet.

Problem 5 :

The angle of the roof on Kaya's doll house is 56°. She built a scale model of the doll house with a scale ratio of 1 : 4, what is the measure of the angle of the roof of the model ?

a) 14°     b) 34°    c) 56°    d)  224°

Solution :

Now, when two figures are similar, then the angle of the two figures are preserved.

This means that the angle of the roof of the model she built will be equal to the actual angle.

Hence, the answer is 56°.

Problem 6 :

In triangle PQR is similar to XYZ.

What is the perimeter of XYZ ?

a) 21 cm     b) 63 cm    c) 105 cm    d)  126 cm

Solution :

Corresponding sides are PQ and XY.

Scale factor = 5 : 30 ==>  1 : 6

Ratio between perimeter and ratio between corresponding sides will be equal.

Perimeter of PQR :

= PQ + QR + RP

= 5 + 6 + 10

= 21

Perimeter of triangle PQR / Perimeter of triangle XYZ = 1 / 6

21 / Perimeter of triangle XYZ = 1 / 6

Doing cross multiplication, we get

21(6) = Perimeter of triangle XYZ.

So, perimeter of triangle XYZ = 126 cm.

Problem 7 :

If the triangles ADE and ABC shown in the figure below are similar, what is the value of x ?

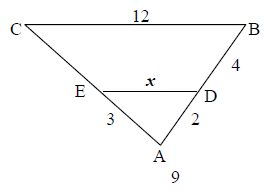
a) 4     b) 5    c) 6    d)  8     e)  10

Solution :

Triangles ADE and ACB

Corresponding sides are AD and AB, AE and AC, ED and CB.

AD/AB = AE/AC = ED/BC

AD/AB = 2/6 ==> 1/3 ---(1)

ED/BC = x/12 ---(2)

(1) = (2)

x/12 = 1/3

x = 12/3

x = 4

Problem 8 :

In the figure given below, the two triangles are similar. What is the value of x ?

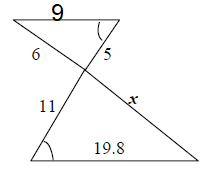
a) 12.4     b) 13.2    c) 14    d)  18.6     e)  22.1

Solution :

Since the sides are similar,

9/19.8 = 5/11 = 6/x

5/11 = 6/x

x = 13.2

Problem 9 :

Mr. Smith is having some photos enlarged for studio. He wants to enlarge a photo that is 5 inches by 7 inches so the dimensions are 3 times larger than the original. How many times larger than the original photo will the area of the new photo be ?

a) 3     b) 6    c) 9    d)  30

Solution :

Length of old photo = 5, Width of old photo = 7

Length of new photo = 15, Width of new photo = 21

Ratio between old to new photo = 1 : 3

Then, ratio between area = 1² : 3²

So, the new photo is 9 times larger.