FINDING AVERAGE RATE OF CHANGE OF COMPOSITE FUNCTION

The graphs of f and g are given below, for each function find the average rate of change of the given interval.

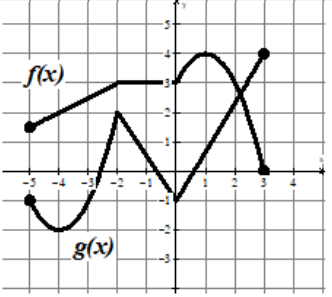
Problem 1 :

h(x) = f(x) + g(x) on [-4, 3]

Solution :

Here a = -4 and b = 3

h(a) = h(-4) = f(-4) + g(-4)

Output of f(-4) is 2, output of g(-4) is -2.

h(-4) = 2 + (-2)

h(-4) = 0

h(b) = h(3) = f(3) + g(3)

Output of f(3) is 0, output of g(3) is 4.

h(3) = 0 + 4

h(3) = 4

Problem 2 :

k(x) = f(g(x)) on [-4, 0]

Solution :

Here a = -4 and b = 0

k(a) = f(g(a)), then k(-4) = f(g(-4))

Output of g(-4) is -2

k(-4) = f(-2)

k(-4) = 3

k(b) = f(g(b)), then k(0) = f(g(0))

Output of g(0) is -1

k(0) = f(-1)

k(0) = 3

Problem 3 :

w(x) = g(f(x)) on [-2, 3]

Solution :

Here a = -2 and b = 3

w(a) = g(f(a)), then k(-2) = g(f(-2))

Output of f(-2) is 3

w(-2) = g(3)

w(-2) = 4

k(b) = g(f(a)), then k(3) = g(f(3))

Output of f(3) is 0

w(0) = g(0)

w(0) = -1

Problem 4 :

The graph shows the depth of water W in a reservoir over a one-year period as a function of the number of days x since the beginning of the year. What was the average rate of change of W between x = 100 and x = 200?

Solution :

(100, 75) and (200, 50)

Average rate of change = (50 - 75)/(200 - 100)

= -25/100

= -1/4