FINDING AVERAGE RATE OF CHANGE OF A FUNCTION
Definition of average rate of change :
The average rate of change between x = a and x = b is the slope of the secant line of the curve between the points x = a and x = b
For the following exercises, find the average rate of change of each function on the interval specified.
Problem 1 :
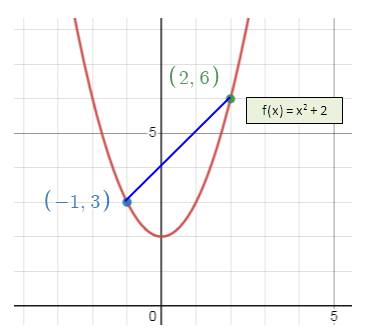
f(x) = x2 + 2 on [-1, 2]
Solution :
Given, f(x) = x2 + 2
a = -1 and b = 2
average rate of change = 1
Problem 2 :
f(x) = 4x2 - 7 on [1, b]
Solution :
Given, f(x) = 4x2 - 7
a = 1 and b = b
average rate of change = 4(b + 1)
Problem 3 :
g(x) = 2x2 - 9 on [4, b]
Solution :
Given, g(x) = 2x2 - 9
a = 4 and b = b
Average rate of change = f(b) - f(a) b - a= f(b) - f(4) b - 4f(4) = 2(4)2 - 9 = 23f(b) = 2(b)2 - 9 = 2b2 - 9Average rate of change = 2b2 - 9 - (23) b - 4= 2b2 - 9 - 23 b - 4= 2b2 - 32 b - 4= 2b2 - 16 b - 4=2b2 - 42 b - 4 =2(b - 4)(b + 4) b - 4= 2(b + 4)average rate of change = 2(b + 4)
For each problem, find the average rate of change of the function over the given interval. You may use the provided graph to sketch the function.
Problem 4 :
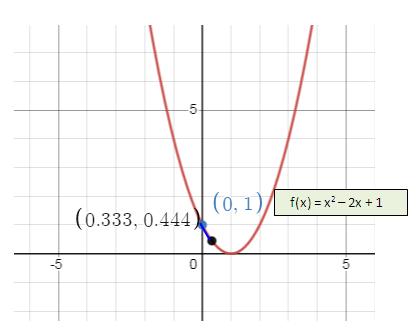
f(x) = x2 - 2x + 1; [0, 1/3]
Solution :
Given, f(x) = x2 - 2x + 1
a = 0 and b = 1/3
Average rate of change = f(b) - f(a) b - a= f13 - f(0) 13 - 0f(0) = (0)2 - 2(0) + 1 = 1f13 = 132 - 213 + 1 = 19 - 23 + 1= 1 - 6 + 99f13= 49Average rate of change = 49 - 1 13 - 0= 4- 9913= -5913= -59 × 31= -53average rate of change = -53
Problem 5 :
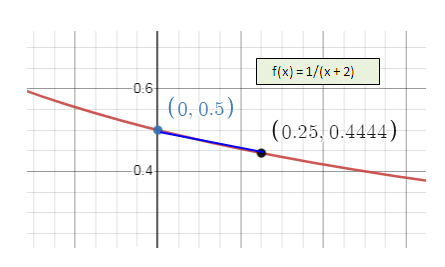
f(x) = 1x + 2; 0, 14
Solution :
Given, f(x) = 1x + 2; 0, 14a = 0 and b = 14Average rate of change = f(b) - f(a) b - a= f14 - f(0) 14 - 0f(0) = 10 + 2 = 12f14 = 114 + 2 = 11 + 84= 194= 49Average rate of change = 49 - 12 14 - 0= 8 - 91814= -11814= -118 × 41= -418average rate of change = -29
Problem 6 :
f(x) = 1x -- 2; -4, -113
Solution :
Given, f(x) = 1x -- 2; -4, -113a = -4 and b = -113Average rate of change = f(b) - f(a) b - a= f-113 - f(-4) -113 + 4 f(-4) = 1-4 - 2 = 1-6f-113 = 1-113 - 2 = 1-11 - 63= 1-173= -317Average rate of change = -317 + 16 -113 + 4= -18 + 17102-11 + 123= -110213= -1102 × 31= -3102average rate of change = -134
Problem 7 :
f(x) = -x2 + 1; [-1, -3/4]
Solution :
Given, f(x) = -x2 + 1
a = -1 and b = -3/4
Given, f(x) = -x2 + 1; -1, -34a = -1 and b = -34Average rate of change = f(b) - f(a) b - a= f-34 - f(-1) -34 + 1 f(-1) = -(-1)2 + 1 = 0f-34 = --342 + 1 = 916 + 1= -9 + 1616= 716 Average rate of change = 716 - 0 -34 + 1= 7 16-3 + 44= 71614= 716 × 41= 2816average rate of change = 74