FINDING AVERAGE OR INSTANTANEOUS RATE OF CHANGE FROM A TABLE
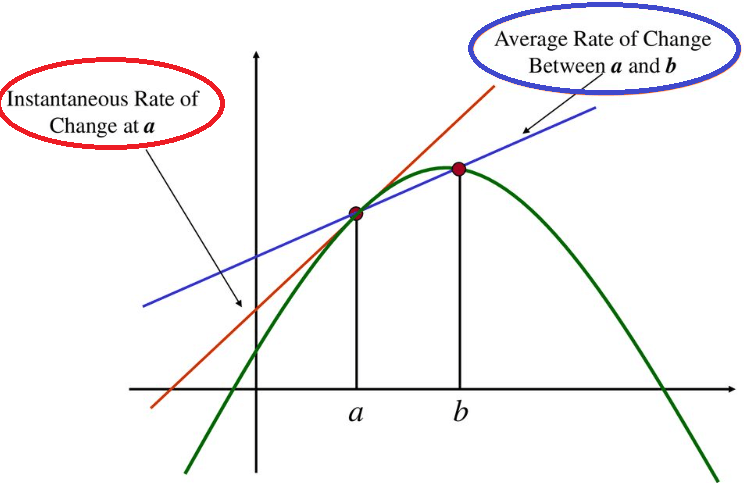
Definition of average rate of change :
The average rate of change between x = a and x = b is the slope of the secant line of the curve between the points x = a and x = b
Instantaneous rate of change :
The instantaneous rate of change at a point x = a is the slope of the tangent line of the curve at the point x = a.
Problem 1 :
Use the following table to find the average rate of change on the given interval.
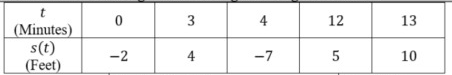
1. [3, 13]
2. 0 ≤ x ≤ 12
3. [3, 4]
Solution:
1. [3, 13]
2. 0 ≤ x ≤ 12
3. [3, 4]
Problem 2 :
The function h(x) is given in the table below. Which of the following choices shows the average rate of change of the function over the interval 2 ≤ x ≤ 6?
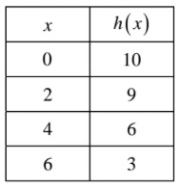
|
|
|
Solution:
So, option (1) is correct.
Problem 3 :
Given the functions g(x), f(x) and h(x) shown below.
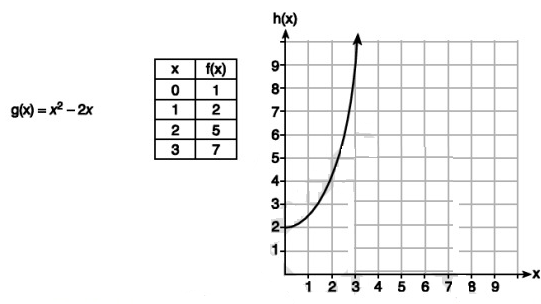
The correct list of functions ordered from greatest to least by average rate of change over the interval 0 ≤ x ≤ 3 is
A. f(x), g(x), h(x) B. h(x), g(x), f(x)
C. g(x), f(x), h(x) D. h(x), f(x), g(x)
Solution:
g(x) = x2 - 2x
For the average rate of change of a function between x = 0 and x = 3.
For the function 'g',
g(x) = x2 - 2x
g(3) = 32 - 2(3)
= 9 - 6
= 3
g(0) = 02 - 2(0)
= 0
h(3) = 9
h(0) = 2
Therefore, order of rate of change from greatest to the least for the given functions will be,
h(x) > f(x) > g(x)
So, option (D) is correct.
Problem 4 :
Use the table below to estimate the value of d'(120). Indicate units of measures.
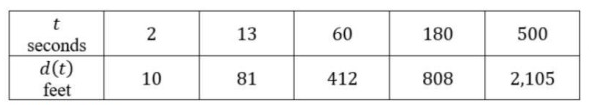
Solution:
d'(120) = 180 - 60
Problem 5 :
Frank is selling lemonade. The function g(t) = (t2 + 4)/2 models the number of glasses he sold, g(t), after t hours. What is the average rate of change between hour 2 and hour 6? Show all work.
Solution:
Problem 6 :
The table shows the average diameter of a person's pupil as a person ages. What is the average rate of change of a person's average pupil diameter from age 30 to 70? Be sure to include units.
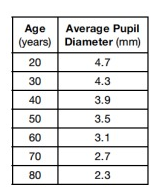
Solution:
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling