FIND THE TURNING POINT OF A PARABOLA

What is turning point of the parabola ?

The vertex is the turning point of the parabola.

There are two types of parabola,

(i) Open upward 

(ii) Open downward

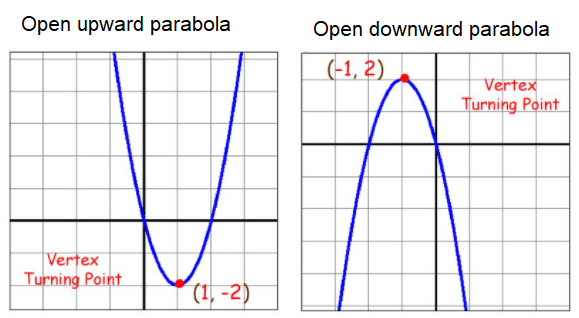
For example,

When we trace the parabola which opens up it will keep on go down after it crosses the turning point or vertex it will go up. That's why we call vertex as turning point.

If the parabola opens up, the vertex represents the lowest point on the graph, or the minimum value of the quadratic function. If the parabola opens down, the vertex represents the highest point on the graph, or the maximum value. 

Find the turning point (vertex) of the following quadratic functions.

Problem 1 :

y = x² - 4x + 2

Solution :

y = x² - 4x + 2

Using completing the square method,

Step 1 :

Since the coefficient of x² is 1, writing the coefficient of x as a multiple of 2.

y = x² - 2⋅x⋅2 + 2

Step 2 :

The middle term looks like 2ab, here b is 2. In order to complete the formula a² - 2ab, we need b²

So,

y = x² - 2⋅x⋅2 + 2² - 2² + 2

To balance +b², we subtract b².

Step 3 :

y = (x - 2)² - 4 + 2

Using algebraic identity for first three terms.

y = (x - 2)² - 2

Here (h, k) is (2, -2).

So, turning point of the parabola is (2, -2)

Method 2 :

Using formula x = -b/2a

y = x² - 4x + 2

a = 1, b = -4 and c = 2

So, the turning point is (2, -2).

Problem 2 :

y = x² + 2x - 3

Solution :

y = x² + 2x - 3

y = x² + 2⋅x⋅1 + 1² - 1² - 3

y = (x + 1)² - 1² - 3

y = (x + 1)² - 4

Turning point is (-1, -4).

Problem 3 :

f(x) = 2x² + 4

Solution :

f(x) = 2x² + 4

Factoring 2, we get

f(x) = 2(x² + 2)

f(x) = 2[(x - 0)² + 2]

f(x) = 2(x - 0)² + 4

Turning point (h, k) is (0, 4).

Problem 4 :

f(x) = 2x² + 4

Solution :

f(x) = -3x² + 1

Factoring 2, we get

f(x) = -3(x² - 1/3)

f(x) = 3[(x - 0)² - (1/3)]

f(x) = 3(x - 0)² - 1

Turning point (h, k) is (0, -1).

Problem 5 :

Using formula to find vertex of the parabola

f(x) = 2x² + 8x - 7

Solution :

Here a = 2, b = 8 and c = -7

So, the turning point us (-2, -15).