FIND THE MISSING VERTICES OF A PARALLELOGRAM

Properties of parallelogram :

• Diagonals bisect each other.

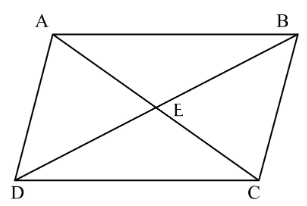
Midpoint of diagonal AC = Midpoint of diagonal DB

Problem 1 :

Show that the points A(3, 1), B(0, -2), C(1, 1) and D(4, 4) are the vertices of a parallelogram ABCD.

Solution:

The given points are A(3, 1), B(0, -2), C(1, 1) and D(4, 4).

Thus, AB = CD = √18 units and BC = AD = √10 units.

Opposite sides are equal.

So, quadrilateral ABCD is a parallelogram.

Problem 2 :

If the points P(a, -11), Q(5, b), R(2, 15) and S(1, 1) are the vertices of a parallelogram PQRS, find the value of a and b.

Solution:

Midpoint of PR = Midpoint of QS

Equating x and y-coordinates, we get

So, the value of a and b are 4 and 3.

Problem 3 :

If three consecutive vertices of a parallelogram ABCD are A(1, -2), B(3, 6) and C(5, 10). Find the fourth vertex D.

Solution:

Let the fourth vertex be D(a, b).

Midpoint of AC = Midpoint of BD

Equating x and y-coordinates, we get

Therefore, the fourth vertex is D(3, 2).

Problem 4 :

If the points A(6, 1), B(8, 2), C(9, 4) and D(p, 3) are the vertices of a parallelogram, taken in order, find the value of p.

Solution:

Midpoint of AC = Midpoint BD

So, the value of p is 7.

Problem 5 :

If the points A(-2, -1), B(a, 0), C(4, b) and D(1, 2) are the vertices of a parallelogram, taken in order, find the value of a and b.

Solution:

Mid point of AC = Mid point of BD

The coordiante of the mid point of a line formed by joining two points (x₁, y₁) and (x₂, y₂) are

By equating x and y-coordinates,

So, the values of a and b are 1 and 3.