FIND THE MISSING INTERIOR AND EXTERIOR ANGLES OF A POLYGON

Find the missing interior and for exterior angles of a given polygon:

Problem 1 :

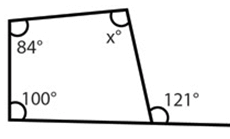
Solution :

y + 121° = 180° (linear pair)

y = 180 - 121

y = 59

x° + 84° + 100° + 59° = 360°

x° + 243 = 360°

x° = 360 - 243

x° = 117

So, the missing angle of x is 117°.

Problem 2 :

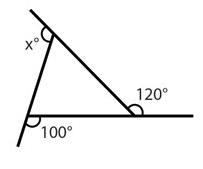
Solution :

The sum of exterior angles of a polygon is 360°.

x° + 100° + 120° = 360°

x° + 220° = 360°

x° = 360 - 220

x° = 140

So, the missing angle of x is 140°.

Problem 3 :

Solution :

The sum of exterior angles of a polygon is 360°.

x° + 95° + 70° + 90° = 360°

x° + 255° = 360°

x° = 360 - 255

x° = 105

So, the missing angle of x is 105°.

Problem 4 :

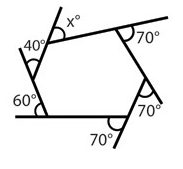
Solution :

The sum of exterior angles of a polygon is 360°.

x° + 70° + 70° + 70° + 60° + 40° = 360°

x° + 310° = 360°

x° = 360 - 310

x° = 50

So, the missing angle of x is 50°.

Problem 5 :

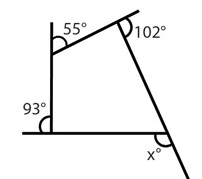
Solution :

The sum of exterior angles of a polygon is 360°.

x° + 93° + 55° + 102° = 360°

x° + 250° = 360°

x° = 360 - 250

x° = 110

So, the missing angle of x is 110°.

Problem 6 :

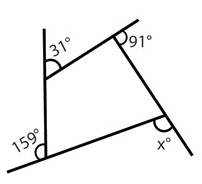
Solution :

The sum of exterior angles of a polygon is 360°.

x° + 159° + 31° + 91° = 360°

x° + 281° = 360°

x° = 360 - 281

x° = 79

So, the missing angle of x is 79°.