FIND THE MISSING ANGLES WITH TRANSVERSAL

Find the angle x in the figure given below. Give your reason.

Example 1 :

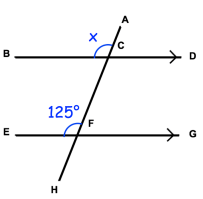
Solution :

∠ACB = ∠CFE

Because they are corresponding angles.

So,

x = 125

Example 2 :

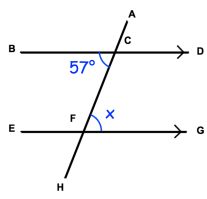
Solution :

∠BCF = ∠CFG

Because they are alternate angles.

So,

x = 57

Example 3 :

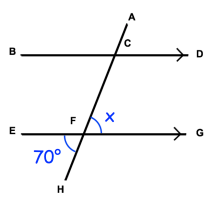
Solution :

∠EFH = ∠CFG

Because they are vertically opposite angles.

So,

x = 70

Example 4 :

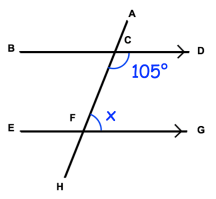
Solution :

∠DCH = ∠CFG

Sum of consecutive interior angle is 180.

x + 105 = 180

x = 180 - 105

x = 75

Example 5 :

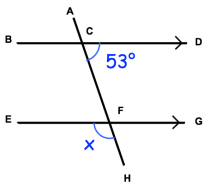
Solution :

∠DCF = ∠EFH

∠DCF = 53

∠CFE + ∠EFH = 180 

53 + x = 180

x = 180 - 53

x = 127

Example 6 :

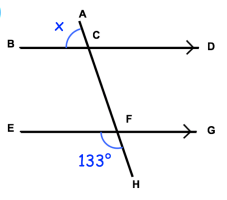
Solution :

∠BCA = ∠DCF (Vertically opposite angles)

∠CFG = ∠EFH (Vertically opposite angles)

∠EFA = ∠BCA = x (Corresponding angles)

∠HFE + ∠EFA = 180

133 + x = 180

x = 180 - 133

x = 47

Example 7 :

Are the lines AB and CD parallel? Explain your answer. 

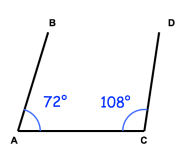
Solution :

By adding the interior angles ,

= ∠BAC + ∠DCA

= 72 + 108

= 180

The sum of interior angles is 180 degree, the angles involving here are parallel.

Example 8 :

Find the missing angle. Give reasons for your answer

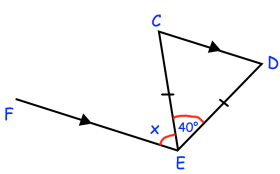
Solution :

Triangle ECD is isosceles triangle.

∠CED + ∠EDC + ∠DCE = 180

∠EDC = ∠DCE

40 + ∠EDC + ∠EDC = 180

Subtract 40 on both sides.

2∠EDC = 180 - 40

2∠EDC = 140

Divide by 2 on both sides.

∠EDC = 70

∠ECD = 70

∠CEF = x

x = 70 (Alternate interior angles)

Example 9 :

Find x.

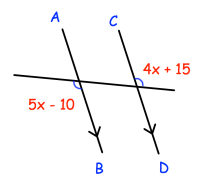
Solution :

5x - 10 = 4x + 15

(Alternate exterior angles)

Subtract 4x on both sides.

5x - 4x - 10 = 15

Add 10 on both sides.

x = 15 + 10

x = 25

Example 10 :

Find x.

Solution :

The angles involving in the problem given above is consecutive interior angles on the same side of the transversal.

x + 22 + 2x - 13  =  180

3x + 9 = 180

3x = 180 - 9

3x = 171

x = 171/3

x = 57