FIND THE LENGTH OF THE DIAGONAL OF EACH SQUARE

The Formula the Area of a Square,

Area = s² sq. units

The Formula the Diagonal of a Square,

d = s√2 units

(where s is the side and d is the diagonal of a square)

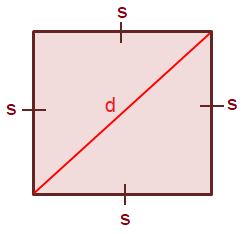
Find the length of the diagonal of each square. Round your answer to the nearest tenth.  

Problem 1 :

Side length = 2 yd

Solution :

Given, side length (s) = 2 yd

Diagonal (d) = s√2

d = 2√2

= 2(1.414)

d = 2 .8 yd

Problem 2 :

Side length = 53 ft

Solution :

Given, side length (s) = 53 ft

Diagonal (d) = s√2

d = 53√2

= 53(1.414)

d = 75 ft

Problem 3 :

Side length = 17.3 in

Solution :

Given, side length (s) = 17.3 in

Diagonal (d) = s√2

d = 17.3√2

= 17.3(1.414)

d = 24 .5 in

Problem 4 :

Side length = 95 yd

Solution :

Given, side length (s) = 95 yd

Diagonal (d) = s√2

d = 95√2

= 95(1.414)

d = 134 .3 yd

Find the length of the diagonal of each square. Round your answer to the nearest tenth.  

Problem 5 :

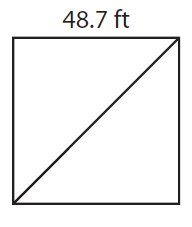
Solution :

By observing the figure,

Side length (s) = 48.7 ft

Diagonal (d) = s√2

d = 48.7√2

= 48.7(1.414)

d = 68 .9 ft

Problem 6 :

Solution :

By observing the figure,

Side length (s) = 32 in

Diagonal (d) = s√2

d = 32√2

= 32(1.414)

d = 45.2 in

Problem 7 :

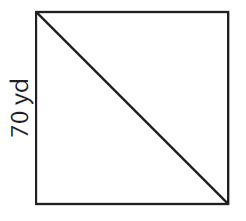
Solution :

By observing the figure,

Side length (s) = 70 yd

Diagonal (d) = s√2

d = 70√2

= 70(1.414)

d = 98.98 yd

Problem 8 :

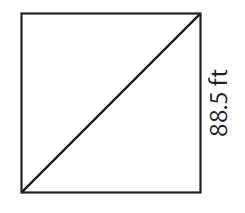
Solution :

By observing the figure,

Side length (s) = 88.5 ft

Diagonal (d) = s√2

d = 88.5√2

= 88.5(1.414)

d = 125 .14 ft

Problem 9 :

The side length of a square is 22 yards. What is the length of the diagonal?

Solution :

Given, side length (s) = 22 yards

Diagonal (d) = s√2

d = 22√2

= 22(1.414)

d = 31.108 yards

So, the length of the diagonal is 31.108 yards.

Problem 10 :

A man waliking at the speed of 4 kmph crosses a square field diagonally in 3 minutes. The area of the field is :

Solution :

Speed of the man = 4 x (5/18) m/sec

= 10/9 m/s

Time taken = 3 x 60

= 180 seconds

Lenght of diagonal = speed x times

= (10/9) x 180

= 200 m

Area of the square field = 1/2 ⋅ (diagonal) ⋅ diagonal

= (1/2) ⋅ 200 ⋅ 200

= 20000 square meter

Problem 11 :

A man walked diagonally across the square lot. approximately, what was the percent saved by not walking along the edges ?

Solution :

Let the side of the square be x meters.

Then AB + BC = 2x

AC = √2x

= 1.414x

Saving n 2x meters = 0.59x m

Saving = (0.59x/2x) ⋅100%

= 30%

So, the required percent is 30% approximately.

Problem 12 :

If the length of the diagonal of a square is 20 cm, then its perimeter must be :

a) 10√2 cm       b) 40 cm      c) 40√2 cm      d) 200 cm

Solution :

Length of diagonal of square = 20 cm

Let x be the side length of square.

x² + x² = 20²

2 x² = 400

x² = 400/2

x² = 200

x = √200

= √(2 x 10 x 10)

= 10√2 cm

Perimeter of square = 4(side length)

= 4 x 10√2

= 40√2 cm

So, option c is correct.

Problem 13 :

The area of a square field 69696 cm². Its diagonal will be equal to 

a) 313.296 m       b) 353.296 m      c) 373.296 m      d) 393.296 m

Solution :

Area of square = 63696

Let x be the side length of square.

x² = 69696

x = √69696

x = 264

Length of diaognal = √2 (side length)

= √2 (264)

= 1.414 (264)

= 373.296 m

So, option c is correct.

Problem 14 :

What will be the length of the diagonal of that square plot whose area is equal to the area of a rectangular plot of the length 45 meters and breadth 40 meters?

a) 42.5 m       b) 60 m      c) insufficient      d) none

Solution :

Length of rectangle = 45 m

breadth = 40 m

Area of rectangular plot = length x breadth

= 45 x 40

= 1800

Area of square = 1800

Let x be the side length of square.

x² = 1800

x = √1800

= √2 x 30 x 30

= 30√2

= 30(1.414)

= 42.4 m

Approximately 42.5 m.

Problem 15 :

The diagonal of a square is 4√2 cm. The diagonal of another square whose area is double that of the first square, is

a)  8 cm     b) 8√2 cm        c)  4√2 cm       d)  16 cm

Solution :

The diagonal of a square = 4√2 cm

Area of the square = a²

Area of first square = √2(4√2)

= 4(2)

= 8 cm²

Double the area of the first square = 8(2)

= 16

Diagonal of another square = 16 cm

So, option d is correct.