FIND THE LENGTH OF A MISSING SIDE OF A RIGHT TRIAGLE

In a right angled triangle, with hypotenuse c and legs a and b.

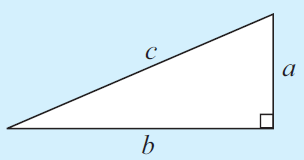
c² = a² + b²

Note :

The hypotenuse is always the longest side and it is opposite the right angle.

• If we know the lengths of any two sides of the triangle then we can calculate the length of the third side.
• If we know the lengths of three sides then we can determine whether it is a right triangle or not.

Find x.

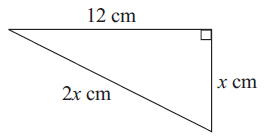
Problem 1 :

Solution :

The side which is opposite to right angle is 2x.

Hypotenuse = 2x

Using Pythagorean theorem :

(2x)² = 12² + x²

4x² = 144 + x²

Subtract x² on both sides, we get

3x² = 144

Divide by 3 on both sides, we get

x² = 144/3

x² = 48

x = √48

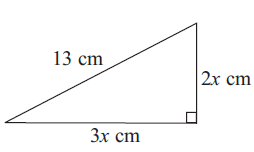
Problem 2 :

Solution :

The side which is opposite to right angle is 13.

Hypotenuse = 13

Using Pythagorean theorem :

(13)² = (3x)² + (2x)²

169 = 9x² + 4x²

169 = 13x²

x² = 169/13

x² = 13

x = √13

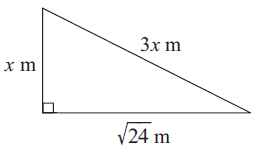
Problem 3 :

Solution :

The side which is opposite to right angle is 3x.

Hypotenuse = 3x

Using Pythagorean theorem :

(3x)² = x² + (√24)²

9x² = x² + 24

Subtracting x² on both sides, we get

8x² = 24

Dividing by 8 on both sides, we get

x² = 3

x = √3

Problem 4 :

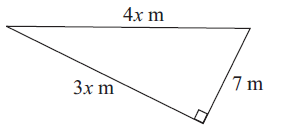
Solution :

The side which is opposite to right angle is 4x.

Hypotenuse = 4x

Using Pythagorean theorem :

(4x)² = (3x)² + 7²

16x² = 9x² + 49

Subtracting 9x² on both sides.

7x² = 49

Dividing by 7 on both sides, we get

x² = 49/7

x² = 7

x = √7

Problem 5 :

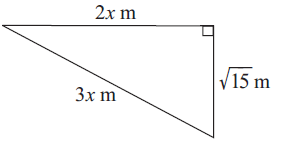
Solution :

The side which is opposite to right angle is 3x.

Hypotenuse = 3x

Using Pythagorean theorem :

(3x)² = (2x)² + √15²

9x² = 4x² + 15

Subtracting 4x² on both sides.

5x² = 15

Dividing by 5 on both sides, we get

x² = 15/5

x² = 3

x = √3

Problem 6 :

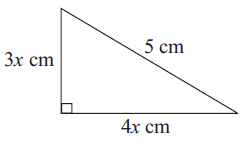
Solution :

The side which is opposite to right angle is 5.

Hypotenuse = 5

Using Pythagorean theorem :

5² = (3x)² + (4x)²

25 = 9x² + 16x²

25 = 25x²

x² = 1

x = 1