FIND THE BASE AND HEIGHT OF A PARALLELOGRAM
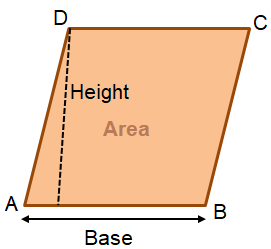
In the Above figure, ABCD is a Parallelogram.
The formula for Area of a Parallelogram,
Area = Base x height
If given area and base, then the formula for height
Height = Area / Base
If given area and height, then the formula for base
Base = Area / Height
Find the base or height of each parallelogram.
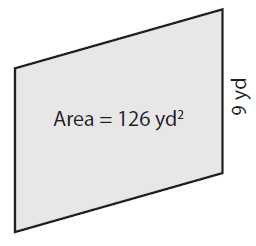
Problem 1 :
Solution :
Given, Area = 126 yd² and Base = 9 yd
Area of a parallelogram = Base x height
To find the height,
Height = Area / Base
Height = (126 yd²) / 9 yd
= 14 yd
So, the height of a parallelogram is 14 yd.
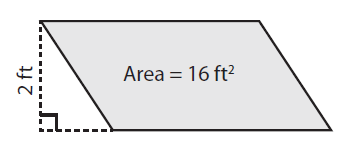
Problem 2 :
Solution :
Given, Area = 16 ft² and Height = 2 ft
Area = Base x Height
To find the base,
Base = Area / Height
Base = (16 ft²) / 2 ft
= 8 ft
So, the base of a parallelogram is 8 ft.
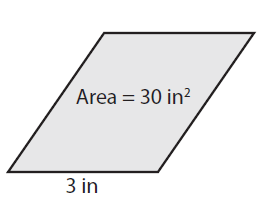
Problem 3 :
Solution :
Given, Area = 30 in² and Base = 3 in
Area = Base x height
To find the height,
Height = Area / Base
Height = (30 in²) / 3 in
= 10 in
So, the height of a parallelogram is 10 in.
Problem 4 :
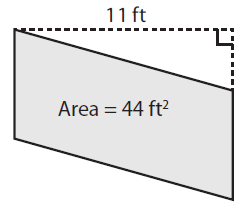
Solution :
Given, Area = 44 ft² and Height = 11 ft
To find the base,
Base = Area / height
Base = (44 ft²) / 11 ft
= 4 ft
So, the base of a parallelogram is 4 ft.
Problem 5 :

Solution :
Given, Area = 160 in² and Base = 10 in
To find the height,
Height = Area / base
Height = (160 in²) / 10 in
= 16 in
So, the height of a parallelogram is 16 in.
Problem 6 :
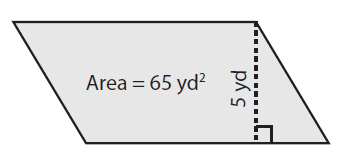
Solution :
Given, Area = 65 yd² and Height = 5 yd
To find the base,
Base = Area / height
Base = (65 yd²) / 5 yd
= 13 yd
So, the base of a parallelogram is 13 yd.
Problem 7 :
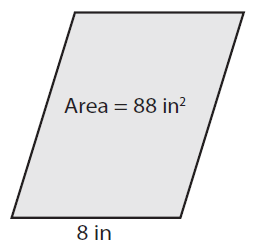
Solution :
Given, Area = 88 in² and Base = 8 in
To find the height,
Height = Area / base
Height = (88 in²) / 8 in
= 11 in
So, the height of a parallelogram is 11 in.
Problem 8 :
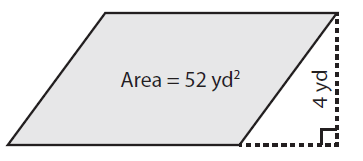
Solution :
Given, Area = 52 yd² and Height = 4 yd
To find the base,
Base = Area / height
Base = (52 yd²) / 4 yd
= 13 yd
So, the base of a parallelogram is 13 yd.
Problem 9 :
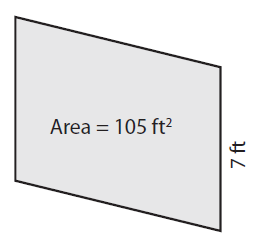
Solution :
Given, Area = 105 ft² and Base = 7 ft
To find the height,
Height = Area / base
Height = (105 ft²) / 7 ft
= 15 ft
So, the height of a parallelogram is 15 ft.
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling