FIND RADIUS OF CYLINDER OF  GIVEN SURFACE AREA

Work out the radius of each cylinder below

Problem 1 :

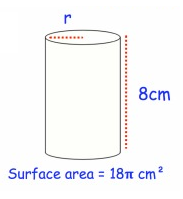
Solution :

By observing the figure,

Radius r = ?

Height h = 8 cm

Surface area A = 18π cm²

Surface area A= 2πr(h + r)

18π = 2πr(8 + r)

Divide each side by 2π.

18π/2π = (2πr(8 + r))/2π

9 = r(8 + r)

9 = 8r + r²

 r² + 8r – 9 = 0

(r + 9)(r - 1) = 0

r = 1 cm

Problem 2 :

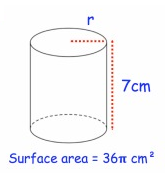
Solution :

By observing the figure,

Radius r = ?

Height h = 7 cm

Surface area A = 36π cm²

Surface area A= 2πr(h + r)

36π = 2πr(7 + r)

Divide each side by 2π.

36π/2π = (2πr(7 + r))/2π

18 = r(7 + r)

18 = 7r + r²

 r² + 7r – 18 = 0

r = 2 cm

Problem 3 :

The radius and height of a cylinder are 5 : 7 and volume is 550 cm³. Find the total surface area.

Solution :

Radius = 5x and height = 7x

Volume of cylinder = 550 cm³

πr²h = 550

Radius = 5(1) ==> 5

height = 7(1) ==> 7

Total surface area of cylinder = 2πr(h + r)

= 2π . 5(7 + 5)

= 10π (12)

= 120π cm²

Problem 4 :

The circumference of the base of a right circular cylinder is 220 cm. If the height of the cylinder is is 2 m, find the total  surface area of cylinder.

Solution :

Circumference of the base of cylinder = 220 cm

2πr = 220

2. (22/7) . r = 220

r = 220 . (7/22) . (1/2)

r = 35

h = 2 m

Curved surface area of cylinder = 2πr(h + r)

Problem 5 :

A roller of diameter 70 cm and the length 2 m is rolling on the ground. What is the area by the roller in 50 revolutions ?

Solution :

Radius of the roller = 70 cm

height of the roller = 2 m ==>  200 cm

Area covered in revolution = 2πrh

= 2 . (22/7) . 70 . 200

= 88000 cm²

= 8.8 m²

Area covered in 50 revolution = 8.8 (50)

= 440 m²