FIND RADIUS OF CONE WHEN GIVEN SURFACE AREA

Find length of radius of the following cones given below.

Problem 1 :

Solution :

Total surface area = πr(l + r)

πr(l + r) = 36π

radius(r) = x and slant height (l) = 5 cm

xπ(5 + x) = 36π

5x + x² = 36

x² + 5x - 36 = 0

(x + 9) (x - 4) = 0

x = -9 and x = 4

So, the required radius is 4 cm.

Problem 2 :

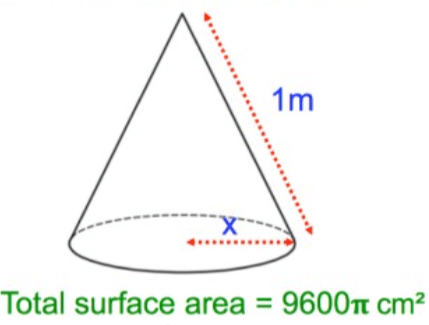
Solution :

Total surface area = πr(l + r)

πr(l + r) = 9600π

radius(r) = x and slant height (l) = 1 m = 100 cm

xπ(100 + x) = 9600π

100x + x² = 9600

x² + 100x  - 9600 = 0

(x + 160)(x - 60) = 0

x = -160 and x = 60

So, the radius is 60 cm.

Problem 3 :

The cylinder and cone has the same surface area. Express L in terms of x.

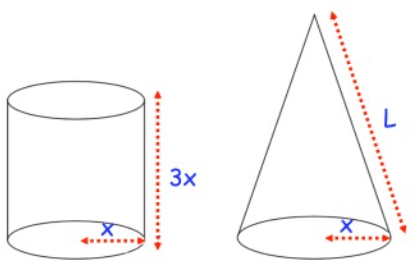
Solution :

Surface area of cylinder = 2πr(h + r)

Surface area of cone = πr(l + r)

height of cylinder = 3x and slant height of cone = l

2πx(3x + x) = πx(l + x)

2x(4x) = x(l + x)

8x² = lx + x²

Subtracting x² on both sides.

7x² = lx

l = 7x

Problem 4 :

A cone and cylinder are joined to make a solid

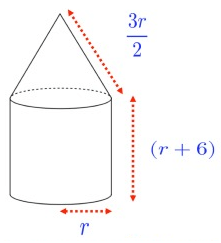
Find the total surface area of the solid.

Solution :

Total surface area of the figure given above = 

lateral surface area of cylinder + lateral surface area of cone

= 2πrh + πrl

= πr(2h + l)

= πr(2(r+6) + (3r/2))

= πr(2r + 12 + (3r/2))

= πr((4r + 24 + 3r)/2)

= πr((7r + 24)/2)