FIND QUADRATIC EQUATION WHEN ROOTS ARE GIVEN

Problem 1 :

Form the equation whose roots are 1 and -5.

Solution :

Let the given roots be α and β

 Sum of the roots = α + β 

Product of roots = αβ

α = 1, β = -5 

α + β = 1 + (-5) = 1 – 5 = -4

αβ = 1 × (-5) = -5

x² – (α + β)x + αβ = 0

x² – (-4)x + (-5) = 0

x² + 4x - 5 = 0

So, the equation is x² + 4x - 5 = 0.

Problem 2 :

Form the equation whose roots are

(√3 - 2)/2 and (√3 + 2)/2

Solution :

Roots are (√3 - 2)/2 and (√3 + 2)/2

Sum of the roots = α + β 

Product of roots = αβ

α = (√3 - 2)/2, β = (√3 + 2)/2

x² - √3 x + (-1/4) = 0

4x² - √3 x -1 = 0

Problem 3 :

If 1 – i and 1 + i are the roots of the equation

x² + ax + b = 0

where a, b ∈  r, then find the values of a and b.

Solution :

α = 1 - i and β = 1 + i

Creating quadratic equation,

α + β = 1 - i + 1 + i ==> 2

α β = (1 - i) (1 + i) ==> 1 - i² ==> 1 - (-1) ==> 2

x² - 2x + 2 = 0

Comparing the given equation with 

x² + ax + b = 0

a = -2 and b = 2

Problem 4 :

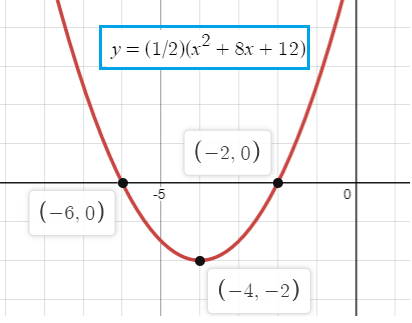
The roots of a quadratic equation are -2 and -6. The minimum point of the graph of its related function is at (-4, -2). Sketch the graph of the function.

Solution :

α = -2 and β = -6

α + β = -2 - 6 ==> -8

α β = -2 (-6) ==> 12

The quadratic function will be,

y = a(x² - (-8)x + 12)

y = a(x² + 8x + 12)

Since the quadratic function is having a minimum point (-4, -2), the will pass through this point.

-2 = a((-4)² + 8(-4) + 12)

-2 = a(16 - 32 + 12)

-2 = a(-4)

a = 1/2

By applying the value of a, we get

y = (1/2)(x² + 8x + 12)

So, the required quadratic function is

y = (1/2)(x² + 8x + 12)

Problem 5 :

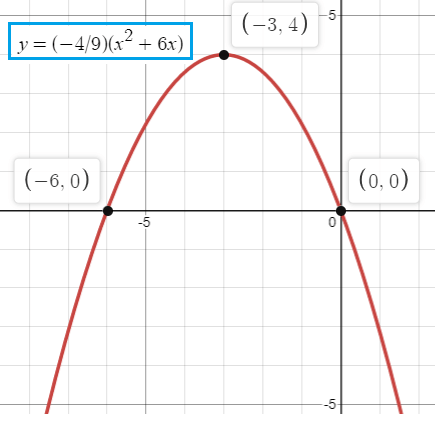
The roots of a quadratic equation are -6 and 0. The minimum point of the graph of its related function is at (-3, 4). Sketch the graph of the function.

Solution :

α = -6 and β = 0

α + β = -6 + 0 ==> -6

α β = -6 (0) ==> 0

The quadratic function will be,

y = a(x² - (-6)x + 0)

y = a(x² + 6x)

Since the quadratic function is having a minimum point (-3, 4), the will pass through this point.

4 = a((-3)² + 6(-3))

4 = a(9 - 18)

4 = a(-9)

a = -4/9

By applying the value of a, we get

y = (-4/9)(x² + 6x)

So, the required quadratic function is

y = (-4/9)(x² + 6x)