FIND EQUATION OF PARABOLA WITH VERTEX AND FOCUS

Find the equation of the parabola that satisfies the given conditions.

Problem 1 :

Vertex (0, 0) and focus (0, -2)

Solution :

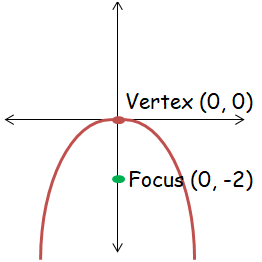
From the picture above, the parabola is symmetric about y-axis and it opens down.

Distance between vertex and focus

= √(x₂ - x₁)² + (y₂ - y₁)²

(0, 0) and (0, -2)

= √(0 - 0)² + (0 + 2)²

= √4

= 2

(y - 0)² = 4a(x - 0)

Applying the value of a, we get

y² = 4(2)x

y² = 8x

So, the required equation of parabola is y² = 8x.

Problem 2 :

Vertex (3, 2) and focus (3, 6)

Solution :

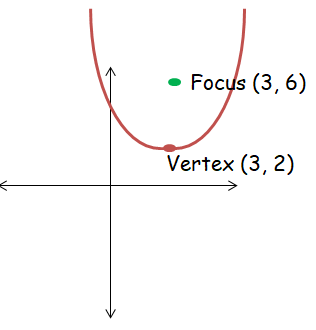
From the picture above, the parabola is symmetric about y-axis and it is open upward.

Distance between vertex and focus

= √(x₂ - x₁)² + (y₂ - y₁)²

(3, 2) and (3, 6)

= √(3 - 3)² + (2 - 6)²

= √(-4)²

a = 4

(y - 2)² = 4a(x - 3)

Applying the value of a, we get

(y - 2)² = 4(4)(x - 3)

(y - 2)² = 16(x - 3)

So, the required equation of parabola is (y - 2)² = 16(x - 3)

Problem 3 :

Find the vertex and focus of the parabola. 

(y - 2)² + 16(x - 3) = 0

Solution :

(y - 2)² + 16(x - 3) = 0

(y - 2)² = -16(x - 3)

Comparing with (y - k)² = -4a(x - h)

4a = 16

a = 16/4

a = 4

The given parabola is symmetric about x-axis and open leftward parabola.

(h, k) => (3, 2)

Focus (h - a, k) ==> (3 - 4, 2)

(-1, 2)

Problem 4 :

Find the standard form of the equation of the parabola with the given characteristic and vertex at the origin. focus: (0, 7)

Solution :

Vertex is at origin. Focus (0, 7)

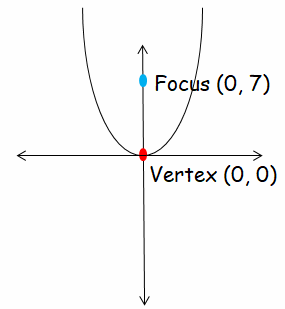
From the picture drawn above, we come to know that the parabola is symmetric about y-axis and open upward.

(y - k)² = 4a(x - h)

Vertex is at (0, 0)

(y - 0)² = 4a(x - 0)

y² = 4ax

Distance between vertex and focus = 7

y² = 4(7)x

y² = 28x

So, the required equation will be y² = 28x.

Problem 5 :

Find the equation of the parabola with vertex at (5, 4) and focus at (-3, 4).

Solution :

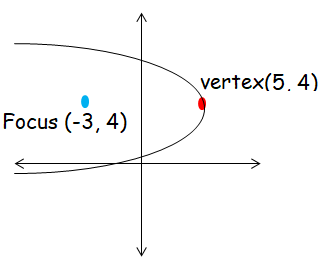
From the picture above, the parabola is symmetric about y-axis and it is open upward.

Distance between vertex and focus

= √(x₂ - x₁)² + (y₂ - y₁)²

V(5, 4) and F(-3, 4)

= √(-3 - 5)² + (4 - 4)²

= √(-8)²

a = 8

(y - 5)² = 4a(x - 4)

Applying the value of a, we get

(y - 5)² = 4(8)(x - 4)

(y - 5)² = 32(x - 4)

So, the required equation of parabola is (y - 5)² = 32(x - 4)

Problem 6 :

A cross section of a parabolic reflector is shown in the figure. The bulb is located at the focus and the opening at the focus is 10 cm.

a) Find the equation of parabola

b) Find the diameter of the opening |CD|, 11 cm from the vertex.

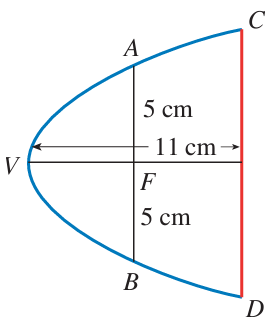
Solution :

The parabola is symmetric about x-axis and open rightward.

(y - k)² = 4a(x - h)

Here F is the focus and the line perpendicular to the axis of symmetry is known as latus rectum.

Length of latus rectum = 10 cm

4a = 10

a = 10/4

a = 5/2

(y - 0)² = 4(5/2)(x - 0)

y² = 10x

Let C be the point (11, y).

y² = 10(11)

y = √110

y = 10.48

CD = 2y

= 2(10.48)

= 20.96

So, the diameter is 20.96 cm.

Problem 7 :

Find the equation of the parabola. Then find focus and directrix.

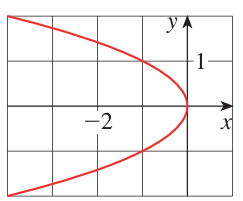
Solution :

By observing the figure, the parabola is symmetric about x-axis and open right ward.

(y - k)² = -4a(x - h)

Vertex is at (0, 0) any point on the parabola is at (-1, 1)

y² = -4ax -----(1)

The parabola is passing through the point (-1, 1)

1² = -4a(-1)

1 = 4a

Applying the value of 4a in (1), we get

y² = -x

Focus (-a, 0) ==> (-1/4, 0)

Equation of directrix ==> x = a

x = 1/4

Problem 8 :

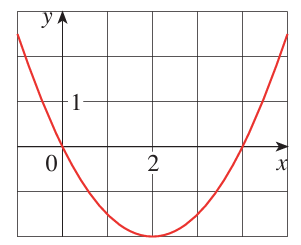
Solution :

By observing the figure, the parabola is symmetric about y-axis and open up ward.

(x - h)² = 4a(y - k)

Vertex is at (2, -2) any point on the parabola is at (4, 0)

(x - 2)² = 4a(y - (-2))

(x - 2)² = 4a(y + 2) ----(1)

The parabola is passing through the point (4, 0)

(4 - 2)² = 4a(0 + 2)

4 = 8a

a = 4/8

a = 1/2

Applying the value of a in (1), we get

(x - 2)² = 4(1/2)(y + 2) 

(x - 2)² = 2(y + 2) 

Focus (h, k + a) ==> (2, -2 + 1/2)

= (2, -3/2)

Equation of directrix ==> y = k - a

y = -2 - (1/2)

y = -5/2