FIND EQUATION OF PARABOLA FROM VERTEX AND POINT

Use the description of the parabola to write its equation in vertex form.

Problem 2 :

A parabola with vertex (0, -3) ; passes through (3, 0)

Solution :

The vertex is (0, -3), so, the equation is f(x) = a(x – 0)² - 3. Find a by substituting the coordinates of another point on the parabola, such as (3, 0).

0 = a(3 – 0)² - 3

0 = a(3)² - 3

0 = 9a - 3

9a = 3

Dividing 9 on both sides.

9a/9 = 3/9

a = 1/3

f(x) = (1/3)(x – 0)² - 3

The equation for the parabola is f(x) = (1/3)x² - 3.

Problem 3 :

A parabola with vertex (-1, 4) ; passes through (-2, 3)

Solution :

The vertex is (-1, 4), so, the equation is f(x) = a(x + 1)² + 4. Find a by substituting the coordinates of another point on the parabola, such as (-2, 3).

3 = a(-2 + 1)² + 4

3 = a(-1)² + 4

3 = a + 4

Substituting 4 on both sides.

3 – 4 = a + 4 – 4

-1 = a

The equation for the parabola is f(x) = -1(x + 1)² + 4.

For each graph, write a quadratic function in vertex form and standard form.

Problem 4 :

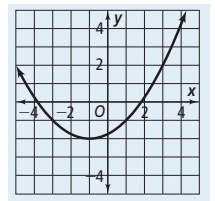
Solution :

Quadratic function in vertex form :

y = a(x – h)² + k

Vertex v = (h, k)

h = -1, k = -2

(h, k) = (-1, -2)

(x, y) = (3, 2)

y = a(x + 1)² – 2

2 = a(3 + 1)² – 2

2 = a(4)² – 2

2 = 16a – 2

Adding 2 on both sides.

2 + 2 = 16a – 2 + 2

4 = 16a

Dividing 4 on both sides.

4/4 = 16a/4

1 = 4a

1/4 = a

Vertex form :

y = (1/4)(x + 1)² – 2

Standard form :

y = 1/4(x² + 2x + 1) – 2

y = 1/4x² + 2/4x + 1/4 – 2

y = (x² + 2x + 1 – 8)/4

y = (1/4) (x² + 2x – 7)

Problem 5 :

Solution :

Quadratic function in vertex form :

y = a(x – h)² + k

Vertex v = (h, k)

h = 2, k = 3

(h, k) = (2, 3)

(x, y) = (4, 7)

y = a(x - 2)² + 3

7 = a(4 - 2)² + 3

7 = a(2)² + 3

7 = 4a + 3

Subtracting 3 on both sides.

7 – 3 = 4a + 3 – 3

4 = 4a

Dividing 4 on both sides.

4/4 = 4a/4

1 = a

Vertex form :

y = (x - 2)² + 3

Standard form :

y = x² – 2(x)(2) + 2² + 3

= x² – 4x + 4 + 3

= x² – 4x + 7

Problem 6 :

What is the equation for the parabola shown in the graph ?

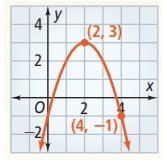
Solution :

The vertex is (2, 3), so, the equation is f(x) = a(x – 2)² + 3. Find a by substituting the coordinates of another point on the parabola, such as (4, -1).

-1 = a(4 – 2)² + 3

-1 = a(2)² + 3

-4 = 4a

a = -1

The equation for the parabola is f(x) = -(x – 2)² + 3.