EXPRESS THE FOLLOWING RATIOS IN SIMPLEST FORM

What is a ratio ?

A ratio is a comparison made by dividing the size of two or more quantities of the same type.

The measurements of quantities should be in the same units.

To express the given ratio in a simplest form, we follow the below steps.

If the given quantities are large integers, we divide both numerator and denominator by HCF of it.
If the given quantities are decimal, we have to multiply both numerator and denominator by 10 to get the integers.
If the given quantities are mixed fractions, we convert them into improper fractions and simplify.

Problem 1 :

a) 2 : 4

b) 56 : 14

c) 15 : 25

d) 0.4 : 0.5

e) 0.2 : 0.6

f) 0.03 : 0.15

g) 1/5 : 4/5

h) 5/4 : 1/4

i) 1/3 : 2

j) 3 : 1/2

Solution :

= 2 : 4

HCF(2, 4) is 2.

= (2/2) : (4/2)

S.F = 1 : 2

= 0.03 : 0.15

= (0.03×100) : (0.15×100)

= 3 : 15

HCF(3, 15) is 3.

= 3/3 : 15/3

S. F = 1 : 5

= 56 : 14

HCF(56, 14) is 14.

= (56/14) : (14/14)

S. F = 4 : 1

= 1/5 : 4/5

= (1/5×5) : (4/5×5)

S.F = 1 : 4

= 15 : 25

HCF(15, 25) is 5.

= (15/5) : (25/5)

S. F = 3 : 5

= 5/4 : 1/4

= (5/4×4) : (1/4×4)

S. F = 5 : 1

= 0.4 : 0.5

= (0.4×10) : (0.5×10)

S. F = 4 : 5

= 1/3 : 2

= (1/3×3) : (2×3)

S. F = 1 : 6

= 0.2 : 0.6

= (0.2×10) : (0.6×10)

= 2 : 6

HCF(2, 6) is 2.

= (2/2) : (6/2)

S. F = 1 : 3

= 3 : 1/2

= (3×2) : (1/2×2)

S. F = 6 : 1

Problem 2 :

34 mm to 1 cm

Solution :

Since the given measurements are in different units, first make their units the same.

10 mm = 1 cm

34 mm = 34/10 cm

= 34/10 : 1

= (34/10×10) : (1×10)

= 34 : 10

HCF(34, 10) is 2.

= (34/2) : (10/2)

Simplest Form = 17 : 5

Problem 3 :

2 m to 125 cm

Solution :

Since the given measurements are in different units, first make their units the same.

1 m = 100 cm

2 m = 200 cm

= 200 : 125

HCF(200, 125) is 25.

= (200/25) : (125/25)

Simplest Form = 8 : 5

Problem 4 :

24 seconds to 1 1/2 min

Solution :

= 24 seconds to 1 1/2 min

Converting mixed fraction into improper fraction.

= 24 seconds to 3/2 min

Now, we need to change their units to be the same.

1 min = 60 sec

3/2 min = 3/2 × 60 = 90 sec

= 24 : 90

HCF(24, 90) is 6.

= (24/6) : (90/6)

Simplest Form = 4 : 15

Problem 5 :

2 L to 750 ml

Solution :

= 2 L to 750 ml

Now, we need to change their units to be the same.

1 L = 1000 ml

2 L = 2000 ml

= 2000 : 750

HCF(2000, 750) is 250.

= (2000/250) : (750/250)

Simplest Form = 8 : 3

Problem 6 :

1 week to 3 days

Solution :

= 1 week to 3 days

1 week = 7 days

Simplest Form = 7 : 3

Problem 7 :

4 weeks to 1 year

Solution :

= 4 weeks to 1 year

1 year = 52 weeks

= 4 : 52

HCF(4, 48) is 4.

= (4/4) : (52/4)

Simplest Form = 1 : 13

Problem 8 :

250 g to 1 kg

Solution :

= 250 g to 1 kg

1 kg = 1000 g

= 250 : 1000

= (250/250) : (1000/250)

Simplest Form = 1 : 4

Problem 9 :

1.5 kg to 125 g

Solution :

= 1.5 kg to 125 g

1 kg = 1000 g

1.5 kg = 1500 g

= 1500 : 125

= (1500/125) : (125/125)

S.F = 12 : 1

Problem 10 :

3 min to 45 seconds

Solution :

= 3 min to 45 seconds

1 min = 60 sec

3 min = 3 × 60 = 180 sec

= 180 : 45

= (180/45) : (45/45)

S. F = 4 : 1

Problem 11 :

Express in simplest form the ratio of shaded area: unshaded area

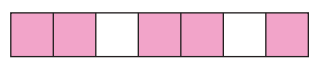	Shaded area : Unshaded area = 5 : 2
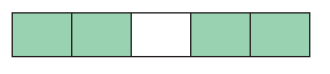	Shaded area : Unshaded area = 4 : 1
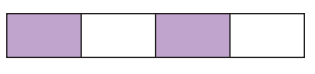	Shaded area : Unshaded area = 2 : 2 = 1 : 1
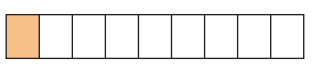	Shaded area : Unshaded area = 1 : 8

EXPRESS THE FOLLOWING RATIOS IN SIMPLEST FORM

Recent Articles

Finding Range of Values Inequality Problems

Solving Two Step Inequality Word Problems

Exponential Function Context and Data Modeling