EQUATIONS OF TANGENTS DRAWN FROM EXTERNAL POINT

Equation of tangent for the parabola from external point :

Equation of tangent for the ellipse from external point :

Equation of tangent for the hyperbola from external point :

Find the equation of the two tangents that can be drawn.

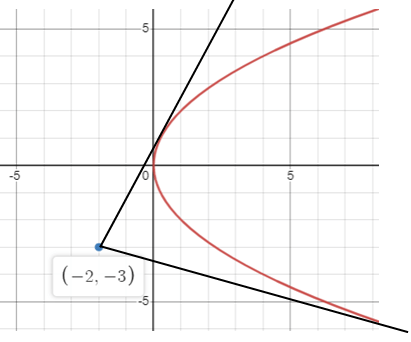
Problem 1  :

From the point (2, -3) to the parabola y² = 4x.

Solution :

Given, y² = 4x

y² = 4ax

4x = 4ax

a = 1

Equation of the tangent to the parabola will be of the form

y = mx + (a/m)

Applying the value of a, we get

y = mx + 1/m

The tangent passes through the point (2, -3)

-3 = 2m + 1/m

-3 = (2m² + 1)/m

-3m = 2m² + 1

2m² - 3m + 1 = 0

So, the equation of the two tangents is

x + 2y + 4 = 0; x + y + 1 = 0.

Problem 2  :

From the point (1, 3) to the ellipse 4x² + 9y² = 36.

Solution :

Given, 4x² + 9y² = 36

Dividing 36 on each sides.

Equation of tangent :

So, the equation of the two tangents is x - 2y + 5 = 0; 5x + 4y - 17 = 0.

Problem 3  :

From the point (1, 2) to the hyperbola 2x² - 3y² = 6.

Solution :

Given, 2x² - 3y² = 6

Dividing 6 on each sides.

Equation of tangent : 

y - y₁ = m(x - x₁)

when m = 1

y - 2 = 1(x - 1)

y - 2 = x - 1

y - 2 - x + 1 = 0

y - x - 1 = 0

Dividing -1 on each sides.

x - y  + 1 = 0

when m = -3

y - 2 = -3(x - 1)

y - 2 = -3x + 3

y - 2 + 3x - 3 = 0

3x + y - 5 = 0

So, the equation of the two tangents is 3x + y - 5 = 0; x - y + 1 = 0.