END BEHAVIOR OF GRAPHS OF POLYNOMIAL FUNCTIONS

Suppose that axⁿ is the dominating term of a polynomial function P of odd degree. (The dominating term is the term of the greatest degree)

Polynomial with Odd Degree

If a > 0, then as x -> ∞, P(x) -> ∞ and as x -> -∞, P(x)  -> -∞. Therefore, the end behavior of the graph is of the type shown below.

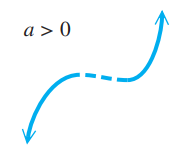
If a < 0, then as x -> ∞, P(x) -> -∞ and as x -> -∞, P(x)  -> ∞. Therefore, the end behavior of the graph is of the type shown below.

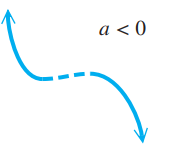
Polynomial with Even Degree

Suppose that axⁿ is the dominating term of a polynomial function P of even degree.

If a > 0, then as | x | -> ∞, P(x) -> ∞. Therefore, the end behavior of the graph is of the type shown below.

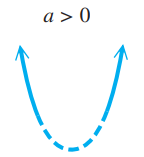
If a < 0, then as | x | -> ∞, P(x) -> -∞. Therefore, the end behavior of the graph is of the type shown below.

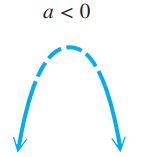
The graphs of the following function are shown.

Problem 1 :

Match the function with its graph.

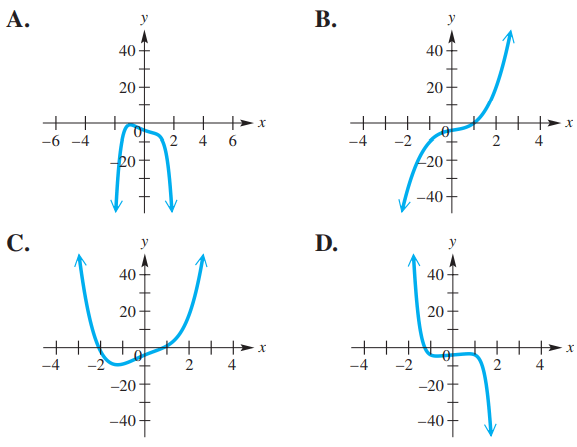
Solution :

 f(x) = x⁴ - x² + 5x - 4

Degree of the polynomial f(x) is 4, it is even.

a = 1  > 0

The function f(x) is even degree with positive leading coefficient on its dominating term. It exactly matches with graph C.

g(x) = -x⁶ + x² - 3x - 4

Degree of the polynomial f(x) is 6, it is even.

a = -1  < 0, then as | x | -> ∞, P(x) -> -∞. Option A is correct.

h(x) = 3x³ - x² + 2x - 4

h(x) is the polynomial of odd degree and a = 3 > 0

When x -> ∞, P(x) -> ∞ and as x -> -∞, P(x)  -> -∞. So, option B is correct.

k(x) = -x⁷ + x - 4

h(x) is the polynomial of odd degree and a = -1 < 0

When | x | -> ∞, P(x) -> -∞. So, option D is correct.

Problem 2 :

Without using a calculator, match each function with the correct graph in choices A–D.

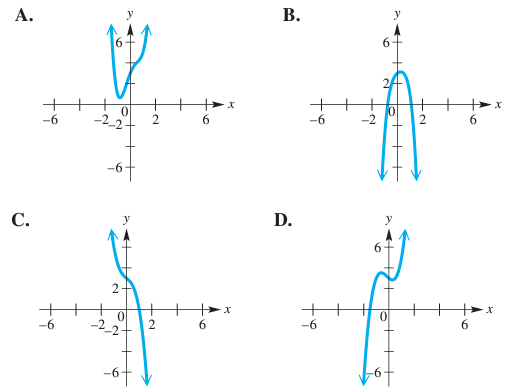
Solution :

f(x) = 2x³ + x² - x + 3

f(x) is the odd degree polynomial, here a = 2 > 0

When x -> ∞, P(x) -> ∞ and as x -> -∞, P(x)  -> -∞. So, option D is correct.

g(x) = - 2x³ - x + 3

g(x) is the odd degree polynomial, here a = -2 < 0

When | x | -> ∞, P(x) -> -∞. So, option C is correct.

h(x) = -2x⁴ + x³ - 2x² + x + 3

It is even degree polynomial. Here a = -2 < 0

When | x | -> ∞, P(x) -> -∞. So, option B is correct.

k(x) = 2x⁴ - x³ - 2x² + 3x + 3

It is even degree polynomial. Here a = 2 > 0

When | x | -> ∞, P(x) -> ∞. So, option A is correct.