DETERMINE WHETHER THE GIVEN PAIR OF FIGURES ARE SIMILAR OR NOT

Problem 1 :

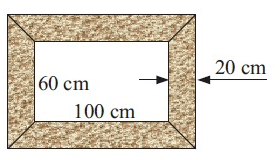
A 20 cm wide picture frame surrounds a painting which is 100 cm by 60 cm. Are the two rectangles shown here similar?

Solution :

Picture frame = 20 cm

Surrounds a painting = 100 cm by 60 cm

100 : 60 = 5 : 3

Inside rectangle has sides in 5 : 3

100 + 60 = 160

20 cm wide picture frame = 160 – 20

= 140

140 : 100 = 7 : 5

Outside rectangle has sides in 7 : 5

And 5 : 3 ≠ 7 : 5

Therefore it is not similar.

Give brief reasons why these figures possess similar triangles:

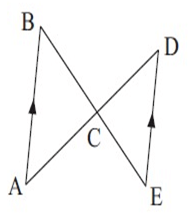
Problem 2 :

Solution :

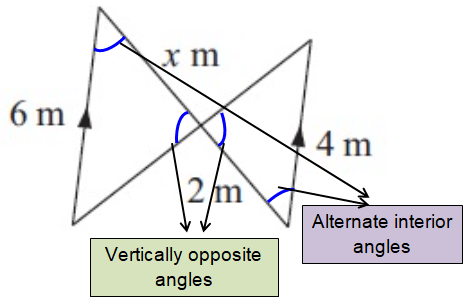
 ∠ABE =  ∠DEB  (Alternate interior angles)

 ∠ACB =  ∠DCE  (Vertically opposite angles)

So, triangles are equiangular and therefore, similar.

Problem 3 :

Solution :

∠PQR =  ∠STR (given)

∠PQT = ∠STP (Alternate interior angles)

∠PRQ = ∠SRT (Vertically opposite angles)

So, triangles are equiangular and therefore, similar.

Problem 4 :

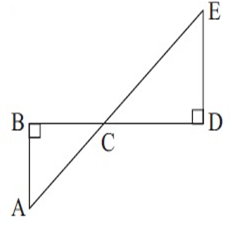
Solution :

Both triangles are right angled.

∠BAE = ∠EDA (Alternate interior angles)

∠BCA = ∠DCE (Vertically opposite angles)

So, triangles are equiangular and therefore similar.

Problem 5 :

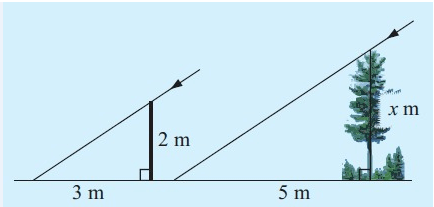
At 4 pm one day the shadow of a pine tree was 5 m long. At the same time a 2 m long broom handle had a shadow which was 3 m long. How high is the pine tree?

Solution :

The shadow of a pine tree = 5 m long

The same time = 2 m long

Broom handle had a shadow = 3 m long

2/3 = x/5

10 = 3x

x = 3.3

So, the pine tree high is 3.3 m.

After establishing similarity, find the unknowns in:

Problem 6 :

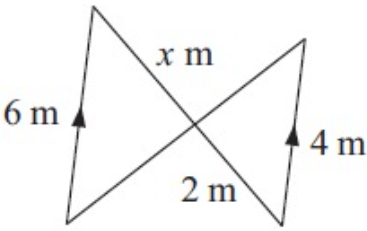
Solution :

6/4 = x/2

3/2 = x/2

x = 3

Problem 7 :

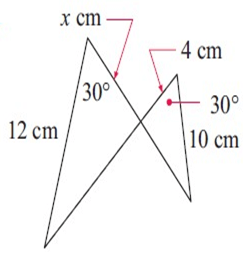
Solution :

12/10 = x/4

6/5 = x/4

x = 4.8

Problem 8 :

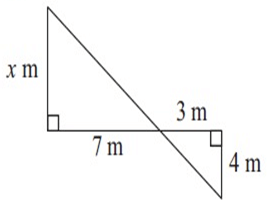
Solution :

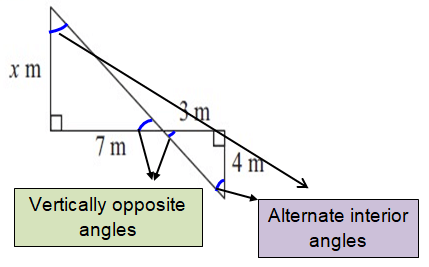
x/4 = 7/3

3x = 28

x = 9 1/3