DETERMINE IF THE SHAPES SHOWN ARE SIMILAR CONGRUENT OR NEITHER

Tell whether the shapes are similar, congruent or neither.

Problem 1 :

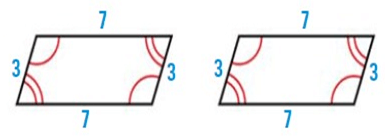
Solution :

As shown, corresponding angles are congruent and corresponding sides are congruent. So, the figures are congruent.

Problem 2 :

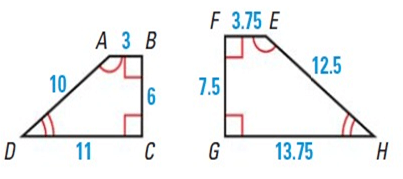
Solution :

As shown, corresponding angles are congruent, but corresponding sides have different lengths. So, the figures are not congruent, but they may be similar.

The figures are similar, if the ratios of the lengths of corresponding sides are equal.

AB/EF = 3/3.75 = 0.8 ----- (1)

BC/FG = 6/7.5 = 0.8 ----- (2)

CD/GH = 11/13.75 = 0.8 ----- (3)

 AD/EH = 10/12.5 = 0.8 ----- (4)

(1) = (2) = (3) = (4)

It has same ratios, so they given shapes are similar.

Problem 3 :

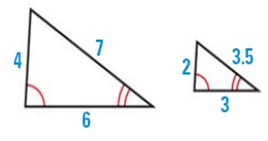
Solution :

As shown, corresponding angles are congruent, but corresponding sides have different lengths. So, the figures are not congruent, but they may be similar.

The figures are similar if the ratios of the lengths of corresponding sides are equal.

4/2 = 2 ----- (1)

7/3.5 = 2 ----- (2)

 6/3 = 2 ----- (3)

(1) = (2) = (3), so the shapes are similar.

Problem 4 :

Solution :

As shown, corresponding angles are congruent and corresponding sides are not congruent. So, the figures are neither.

10/11 = 0.91 ----- (1)

1/2 = 0.5 ----- (2)

So, (1), (2) are neither.

Problem 5 :

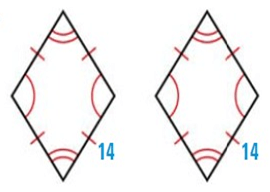
Solution :

As shown, corresponding angles are congruent and corresponding sides are congruent. So, the figures are congruent.

Problem 6 :

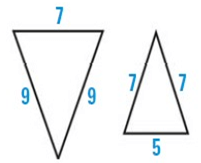
Solution :

As shown, corresponding angles are congruent and corresponding sides are not congruent. So, the figures are neither.

7/5 = 1.4 ----- (1)

9/7 = 1.3 ----- (2)

9/7 = 1.3 ----- (3)

So, (1), (2), (3) are neither.

Problem 7 :

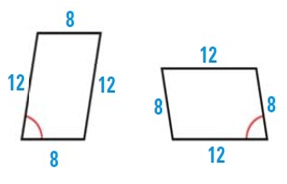
Solution :

As shown, corresponding angles are congruent and corresponding sides are congruent. So, the figures are congruent.

Problem 8 :

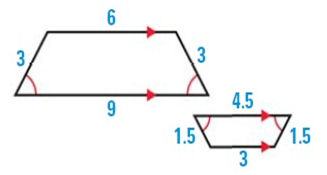
Solution :

As shown, corresponding angles are congruent, but corresponding sides have different lengths. So, the figures are not congruent, but they may be similar.

The figures are similar if the ratios of the lengths of corresponding sides are equal.

6/3 = 2 ----- (1)

3/1.5 = 2----- (2)

3/1.5 = 2 ----- (3)

9/4.5 = 2 ----- (4)

(1) = (2) = (3) = (4), so the shapes are similar.

Problem 9 :

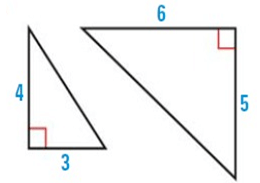
Solution :

As shown, corresponding angles are congruent and corresponding sides are not congruent. So, the figures are neither.

4/5 = 0.8 ----- (1)

6/3 = 2 ----- (2)

So, (1), (2) are neither.

Problem 10 :

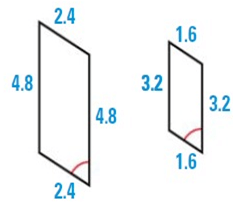
Solution :

As shown, corresponding angles are congruent, but corresponding sides have different lengths. So, the figures are not congruent, but they may be similar.

The figures are similar if the ratios of the lengths of corresponding sides are equal.

2.4/1.6 = 1.5 ------ (1)

4.8/3.2 = 1.5 ----- (2)

4.8/3.2 = 1.5 ----- (3)

2.4/1.6 = 1.5 ----- (4)

(1) = (2) = (3) = (4), so the shapes are similar.