DETERMINE GRAPHICALLY OR ALGEBRAICALLY IF A FUNCTION IS ODD OR EVEN

A Function can be classified as Even, Odd or Neither. This classification can be determined graphically or algebraically.

How to check if the graph is odd ?

The graph will be symmetric with respect to the origin.

In other words :

If you spin the picture upside down about the Origin, the graph looks the same!

How to check if the graph is even ?

The graph will be symmetric with respect to the y-axis.

Graphically Checking If the Function is Odd or Even

Determine graphically using possible symmetry, whether the following functions are even, odd or neither.

Problem 1 :

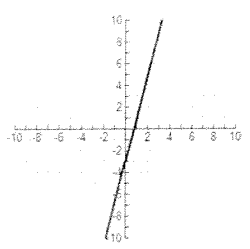
Solution :

The graph is not symmetric about origin, then it is not odd function.

The graph is not symmetric about y-axis, then it is not even function.

So, it is neither.

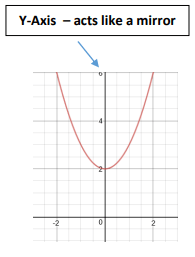
Problem 2 :

Solution :

Here y-axis is acting as a mirror. Clearly it is symmetric about y-axis. Then, it is even function.

Problem 3 :

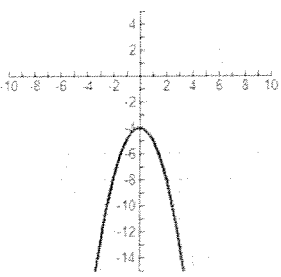
Solution :

Here y-axis is acting as a mirror. Clearly it is symmetric about y-axis. Then, it is even function.

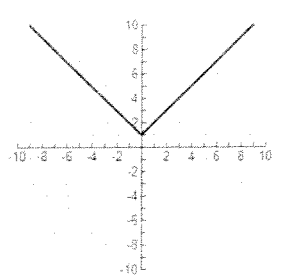
Problem 4 :

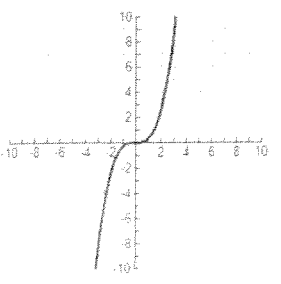
Solution :

The graph is symmetric about origin. So, it is odd function.

Problem 5 :

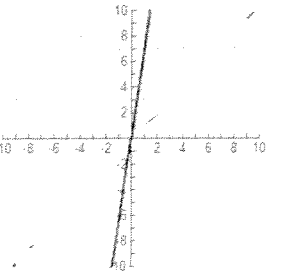
Solution :

The graph is not symmetric about origin, then it is not odd function.

The graph is not symmetric about y-axis, then it is not even function.

So, it is neither.

Problem 6 :

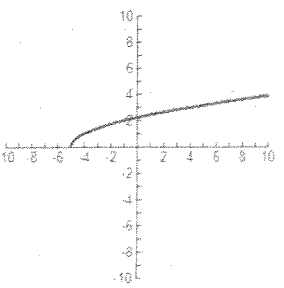
Solution :

The graph is not symmetric about origin, then it is not odd function.

The graph is not symmetric about y-axis, then it is not even function.

So, it is neither.

Problems on Algebraically Checking If It is Odd or Even

Verify algebraically whether each function is even, odd or neither!

Problem 1 :

f(x) = x³ - 6x

Solution:

f(x) = x³ - 6x

Put x = -x

f(-x) = (-x)³ - 6(-x)

= -x³ + 6x

So, it is neither.

Problem 2 :

g(x) = x⁴ - 2x²

Solution:

g(x) = x⁴ - 2x²

Put x = -x

g(-x) = (-x)⁴ - 2(-x)²

= x⁴ - 2x²

So, it is even function.

Problem 3 :

h(x) = x² + 2x + 1

Solution:

h(x) = x² + 2x + 1

Put x = -x

h(-x) = (-x)² + 2(-x) + 1

= x² - 2x + 1

h(-x) ≠ h(x)

So, it is neither.

Problem 4 :

f(x) = x² + 6

Solution:

f(x) = x² + 6

Put x = -x

f(-x) = (-x)² + 6

= x² + 6

f(-x) = f(x)

So, it is even function.

Problem 5 :

g(x) = 7x³ - x

Solution:

g(x) = 7x³ - x

Put x = -x

g(-x) = 7(-x)³ - (-x)

= -7x³ + x

g(-x) ≠ g(x)

So, it is odd function.

Problem 6 :

h(x) = x⁵ + 1

Solution:

h(x) = x⁵ + 1

Put x = -x

h(-x) = (-x)⁵ + 1

= -x⁵ + 1

h(-x) ≠ h(x)

So, it is neither.

Problem 7 :

f(x) = x√(4 - x²)

Solution:

f(x) = x√(4 - x²)

Put x = -x

f(-x) = (-x)√(4 - (-x)²)

= -x√(4 - x²)

So, it is neither.

Problem 8 :

g(x) = x⁴√(1 + x)

Solution:

g(x) = x⁴√(1 + x)

Put x = -x

g(-x) = (-x)⁴√(1 + (-x))

= x⁴√(1 - x)

So, it is neither.

Problem 9 :

h(x) = |x| - 1

Solution:

h(x) = |x| - 1

Put x = -x

h(-x) = |-x| - 1

= x - 1

So, it is even function.

Problem 10 :

Solution:

So, it is even function.