CREATE A QUADRATIC FUNCTION FROM GIVEN ZEROS WORKSHEET

Problem 1 :

Write a quadratic equation in standard form with solutions, x = -3 and x = 4. Use integers for a, b and c.

Solution

Problem 2 :

Write a quadratic equation in standard form with solutions, x = 2/3 and x = -5. Use integers for a, b and c.

Solution

Problem 3 :

Write an equation of the parabola in intercept form y = a(x - p)(x - q) that has x- intercepts of 9 and 1 and passes through (0, -18).

A) y = -1/2(x - 9)(x - 1)              B) y = -1/2(x + 9)(x + 1)

C) y = -2(x - 9)(x - 1)                 D) y = -2(x + 9)(x + 1)

Solution

Problem 4 :

Write an equation of the parabola in intercept form y = a(x - p)(x - q) that has x- intercepts of 12 and -6 and passes through (14, 4).

A) y = 1/10(x - 12)(x + 6)      B) y = 1/10(x + 12)(x - 6)

C) y = 10(x - 12)(x + 6)         D) y = 10(x + 12)(x - 6)

Solution

Problem 5 :

Determine the equation of a quadratic function given zeros x = 4 and point (3, 2).

Solution

Problem 6 :

Use the intercepts and a point on the graph below to write the equation of the function.

Solution

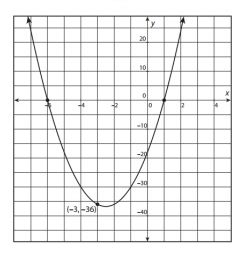
Problem 7 :

Use the intercepts and a point on the graph below to write the equation of the function.

Solution