CONVERSE OF THE PYTHAGOREAN THEOREM FOR CLASSIFYING TRIANGLE

Classify the triangle as acute, right, or obtuse, explain.

Problem 1 :

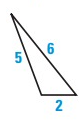
Solution:

Let c represent the length of the longest side of the triangle. 

c² ? a² + b²

6² ? 5² + 2²

36 ? 25 + 4

36 > 29

Because c² is greater than a² + b², the triangle is obtuse.

Problem 2 :

Solution:

Let c represent the length of the longest side of the triangle. 

c² ? a² + b²

17² ? 8² + 15²

289 ? 64 + 225

289 = 289

Because c² is equal to a² + b², the triangle is right.

Problem 3 :

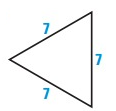
Solution:

Let c represent the length of the longest side of the triangle. 

c² ? a² + b²

72 ? 7² + 7²

49 ? 49 + 49

49 < 98

Because c² is less than a² + b², the triangle is acute.

Use the side lengths to classify the triangle as acute, right, or obtuse.

Problem 4 :

7, 24, 24

Solution:

By Triangle Inequality Theorem, the above set of numbers can represent the side lengths of a triangle.

Compare the square of the length of the longest side with the sum of the squares of the lengths of the two shorter sides. 

c² ? a² + b²

24² ? 7² + 24²

576 ? 49 + 576

576 < 625

Because c² is less than a² + b², the triangle is acute.

Problem 6 :

7, 24, 25

Solution:

By Triangle Inequality Theorem, the above set of numbers can represent the side lengths of a triangle.

Compare the square of the length of the longest side with the sum of the squares of the lengths of the two shorter sides. 

c² ? a² + b²

25² ? 7² + 24²

625 ? 49 + 576

625 = 625

Because c² is equal to a² + b², the triangle is right.

Problem 7 :

7, 24, 26

Solution:

By Triangle Inequality Theorem, the above set of numbers can represent the side lengths of a triangle.

Compare the square of the length of the longest side with the sum of the squares of the lengths of the two shorter sides. 

c² ? a² + b²

26² ? 7² + 24²

676 ? 49 + 576

676 > 625

Because c² is greater than a² + b², the triangle is obtuse.

Determine whether the triangle is acute, right, or obtuse.

Problem 8 :

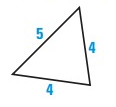
Solution:

Let c represent the length of the longest side of the triangle. 

c² ? a² + b²

5² ? 4² + 4²

25 ? 16 + 16

25 < 32

Because c² is less than a² + b², the triangle is acute.

Problem 9 : 

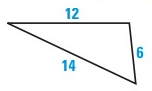
Solution:

Let c represent the length of the longest side of the triangle. 

c² ? a² + b²

14² ? 12² + 6²

196 ? 144 + 36

196 > 180

Because c² is greater than a² + b², the triangle is obtuse.

Problem 10 :

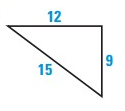
Solution :

Let c represent the length of the longest side of the triangle. 

c² ? a² + b²

15² ? 12² + 9²

225 ? 144 + 81

225 = 225

Because c² is equal to a² + b², the triangle is right.

Problem 11 :

Match the side lengths of a triangle with the best description.

Solution:

1) By Pythagorean Theorem,

11² = 2² + 10² 

121 = 4 + 100

121 > 104

It is obtuse triangle.

2) By Pythagorean Theorem,

7² = 8² + 5² 

49 = 64 + 25

49 < 89

It is acute triangle.

3) 

5, 5, 5

It is equiangular triangle.

4) By Pythagorean Theorem,

10² = 6² + 8² 

100 = 36 + 64

100 = 100

It is right triangle.