CONGRUENT CHORDS AND ARCS

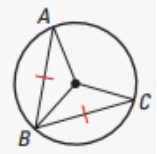
Problem 1:

Find the measure of AB.

Solution :

3x + 54˚ = 5x

5x - 3x = 54˚

2x = 54

x = 27˚

AB = 3(27) + 54

= 81 + 54

AB = 135˚

Problem 2 :

In circle B, CB ≈ BD. Find x if AE = x² and FG = 3x + 4.

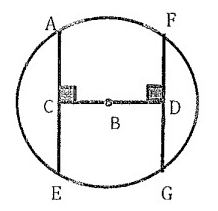
Solution :

In the above diagram, the two chords AE and FG are congruent.

AE = FG

x² = 3x + 4

x² - 3x - 4 = 0

(x + 1) (x - 4) = 0

x = -1 or x = 4

We can use only positive value for x.

So,

x = 4

Problem 3 :

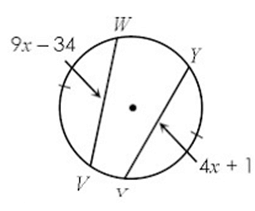
Solution :

In the above diagram, the two chords VW and XY are congruent.

WV = XY

9x - 34 = 4x + 1

9x - 4x = 1 + 34

5x = 35

x = 7

Problem 4 :

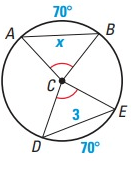
Solution :

Equal chords of a circle subtend equal angles at the center.

∠ACB = ∠DCE

AB = DE

x = 3

Problem 5 :

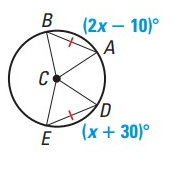
Solution :

AB = DE

2x - 10˚ = x + 30˚

2x - x = 30 + 10

x = 40˚

Problem 6 :

Use the diagram below.

a. Explain why AD ≈ BE.

b. Find the value of x.

c. Find m arc AD and m arc BE.

d. Find m arc BD.

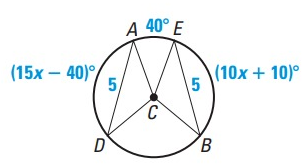
Solution :

a.    

In the diagram above, the two chords AD and BE are congruent.

AD ≈ BE

b.    

15x - 40 = 10x + 10

     15x - 10x = 10 + 40

5x = 50

x = 10

c.    

m arc AD = 15x - 40˚

= 15(10) - 40

= 150 - 40

m arc AD = 110˚

m arc BE = 10x + 10˚

= 10(10) + 10

= 100 + 10

m arc AD = 110˚

d.

m arc BD = 360˚ - (110 + 110 + 40)˚

= 360˚ - 260˚

m arc BD = 100˚

Problem 7:

Solution :

Given, FG = HG = 4

m arc HG = m arc FG =

x + x + 220 = 360

2x = 360 - 220

x = 140/2

x = 7

Problem 8 :

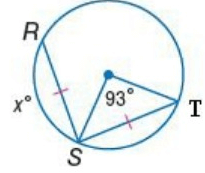
Solution :

RS = ST

So,

x = 93˚

Problem 9 :

Solution :

In the diagram above, the two chords AB and CD are congruent.

AB = CD

5x = 3x + 6

5x - 3x = 6

2x = 6

x = 3