COMPLEMENTARY AND SUPPLEMENTARY ANGLES PRACTICE QUESTIONS
Find the values of unknowns :
Problem 1 :
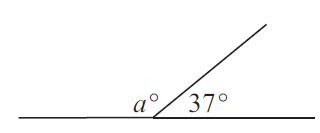
Problem 2 :
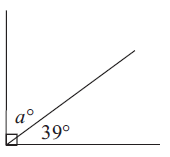
Problem 3 :
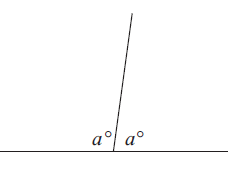
Problem 4 :
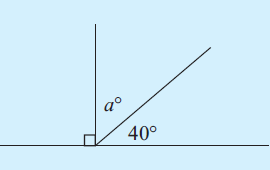
Problem 5 :

Problem 6 :
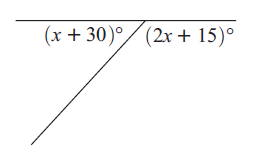
Problem 7 :

Problem 8 :
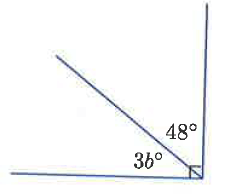
Problem 9 :
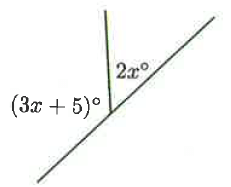
Problem 10 :
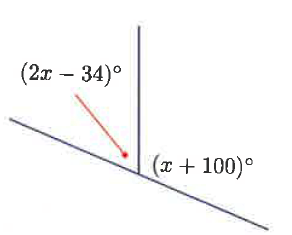
Answer Key
|
1) a = 143 2) a = 51° 3) a = 90 4) a = 50 5) h = 60 |
6) x = 45 7) x = 5 8) b = 14 9) x = 35 10) x = 38 |
Problem 1 :
Angles A and B are complementary. If m∠A = 3x - 8 and m∠B = 5x + 10, what is the measure of each angle ?
Problem 2 :
Angles Q and R are supplementary. If m∠Q = 4x + 9 and m∠R = 8x + 3, what is the measure of each angle ?
Problem 3 :
Find the measure of two complementary angles ∠A and ∠B, if m∠A = 7x + 4 and m∠B = 4x+ 9.
Problem 4 :
The measure of an angle is 44 more than the measure of its supplement. Find the measures of the angles.
Problem 5 :
What are the measures of two complementary angles if the difference in the measures of the two angles is 12.
Problem 6 :
Find the measures of two supplementary angles ∠N and ∠M if the measure of angle N is 5 less than 4 times the measure of angle M.
Problem 7 :
Suppose ∠T and ∠U are complementary angles. Find x, if ∠T = 16x - 9 and ∠U = 4x - 1.
Problem 8 :
Two angles are vertical in relation. One angle is 2y and the other angle is y + 130. Find each angle measure.
Problem 9 :
The measure of two supplementary angles are in the ratio 4 : 2, Find those two angles.
Answer Key
1) m∠A = 25 and m∠B = 65
2) m∠Q = 65 and m∠R = 115
3) m∠A = 53 and m∠B = 37
4) 112 and 68.
5) 39 and 51
6) ∠M = 37 and ∠N = 143
7) 71 and 19
8) 260 and 260
9) 60 and 120
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling