COMPARING UNIT RATE WORD PROBLEMS WORKSHEET

Examine each set of functions and determine which has the greater rate of change, if either. Explain your reasoning.

Problem 1 :

Solution

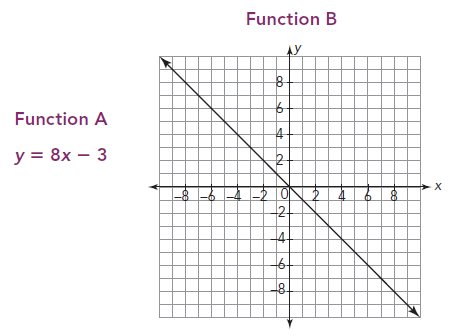
Problem 2 :

Solution

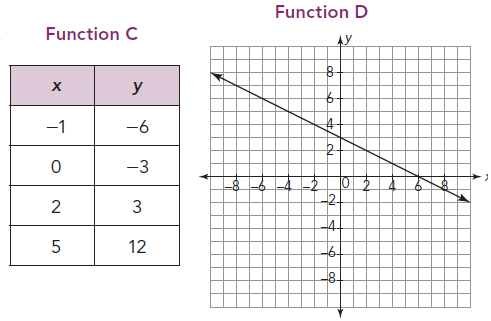
Problem 3 :

Solution

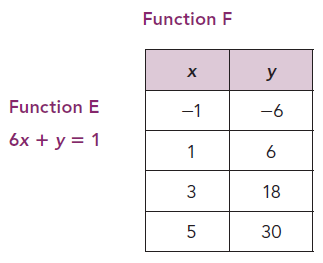
Problem 4 :

Alicia, Cherie, and John had been studying rates of change and were discussing the best way to determine which linear function has the greater rate of change.

Solution

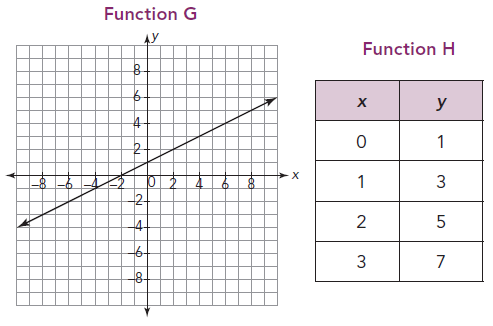
Examine each set of functions and determine which has the greater rate of change, if either. Explain your reasoning.

Problem 5 :

Solution

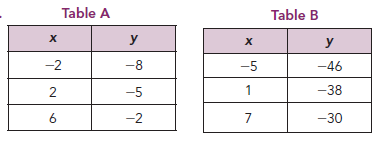
Problem 6 :

Solution

Problem 7 :

Equation A  : 5x + 6y = 60

Equation B  : y = (-1/4)x  - 2

Solution

Problem 8 :

An ice cream shop is choosing a milk delivery service. The Spotted Cow charges $2.80 per gallon, plus a $2 delivery fee. Dairy Farms charges $2.10 per gallon, plus a $10 delivery fee.

Solution

Problem 9 :

Alan and Margot each drive from City A to City B, a distance of 𝟏𝟒𝟕 miles. They take the same route and drive at constant speeds. Alan begins driving at 1:40 p.m. and arrives at City B at 4:15 p.m.

Margot’s trip from City A to City B can be described with the equation 𝒚 = 𝟔𝟒x, where y is the distance traveled in miles and 𝒙 is the time in minutes spent traveling.

Who gets from City A to City B faster?           Solution

Problem 10 :

You have recently begun researching phone billing plans. Phone Company A charges a flat rate of $𝟕𝟓 a month. A flat rate means that your bill will be $𝟕𝟓 each month with no additional costs.

The billing plan for Phone Company B is a linear function of the number of texts that you send that month. That is, the total cost of the bill changes each month depending on how many texts you send.

The table below represents some inputs and the corresponding outputs that the function assigns.

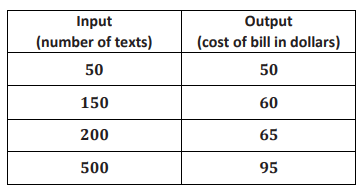
(i)  At what number of texts would the bill from each phone plan be the same?

(ii)  At what number of texts is Phone Company A the better choice?

(iii)  At what number of texts is Phone Company B the better choice?                      Solution

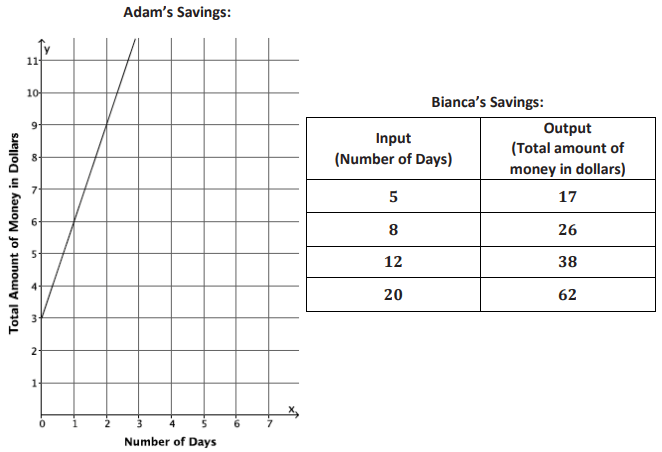
Problem 11 :

Two people, Adam and Bianca, are competing to see who can save the most money in one month. Use the table and the graph below to determine who will save the most money at the end of the month. State how much money each person had at the start of the competition. (Assume each is following a linear function in his or her saving habit.)

Solution