COMPARING AND ORDERING RATIONAL AND IRRATIONAL NUMBERS

How we are comparing and ordering rational and irrational numbers ?

The given numbers may in the form of fraction, percentage, decimals, square roots  etc. We convert the given numbers into decimal and compare them.

Let us understand what are rational and irrational numbers.

Example Problems on Comparing and Ordering Rational and Irrational Numbers

Order the following numbers from least to greatest :

Problem 1 :

135%, 13.5, 8 3/5

Solution :

135%, 13.5, 8 3/5

135/100, 13.5, 43/5

1.35, 13.5, 8.6

Numbers from least to greatest are

1.35, 8.6 and 13.5

So, the numbers from least to greatest is

135% < 8  3/5 < 13.5

Problem 2 :

1/6, 16.7%, 0.16

Solution :

1/6, 16.7%, 0.16

0.166…, 16.7/100, 0.16

0.166…, 0.167, 0.16

So, the numbers from least to greatest is

0.16 < 1/6 < 16.7%.

Order the following numbers from greatest to least :

Problem 3 :

0.42, 4.2%, 2/5

Solution :

0.42, 4.2%, 2/5

0.42, 4.2/100, 0.4

0.42, 0.042, 0.4

So, the numbers from greatest to least is 0.42 > 2/5 > 4.2%.

Problem 4 :

3 1/3, 3.34, 300%

Solution :

3 1/3, 3.34, 300%

10/3, 3.34, 300/100

3.33, 3.34, 3

So, the numbers from greatest to least is

3.34 > 3 1/3 > 300%.

Put the following in ascending order :

Problem 5 :

45%, 1/2, 0.6, 1

Solution :

45%, 1/2, 0.6, 1

45/100, 1/2, 0.6, 1

0.45, 0.5, 0.6, 1

Hence, ascending order is 45% < 1/2 < 0.6 < 1.

Problem 6 :

4, 0.8, 50%, 0

Solution :

4, 0.8, 50%, 0

4, 0.8, 50/100, 0

4, 0.8, 0.5, 0

Hence, ascending order is 0 < 50% < 0.8 < 4.

Put the following numbers in descending order :

Problem 7 :

45%, 1/2, 0.045

Solution :

45%, 1/2, 0.045

45/100, 1/2, 0.045

0.45, 0.5, 0.045

Hence descending order is 1/2 > 45% > 0.045.

Problem 8 :

125%, 1 1/2, 1.26

Solution :

125%, 1 1/2, 1.26

125/100, 3/2, 1.26

1.25, 1.5, 1.26

Hence descending order is 1 1/2 > 1.26 > 125%.

Problem 9 :

Which is least number of the following.

0.4, √0.09 and 1/2

Solution :

0.3 is the least, then √0.09 is least.

Problem 10 :

Which of the following is greater ? or they are same.

a)  √(100 - 64)          b)  √100 - √64

Solution :

They are not same.

a)  √(100 - 64) 

√(100 - 64) = √36 ==> √(6 x 6) ==> 6

b)  √100 - √64

√100 - √64 ==> 10 - 8 ==> 2

So, a is greater.

Problem 11 :

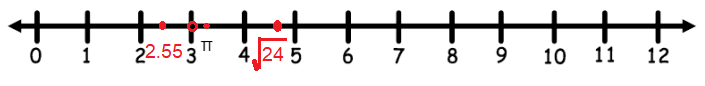
Graph the numbers on the number line.

2.55, 9/5, √24, π, √9

Solution :

2.55 = we cannot do further simplification

9/5 = 1.8

√24 = 4.8

π = 3.14

√9 = √(3 x 3) = 3

Problem 12 :

Write it in order from greatest to least.

10/4, √10, 4.75, √16

Solution :

10/4 = 2.5

√10 = 3.3......

4.75 = 4.75 (already it is a decimal)

√16 = √(4 x 4) ==> 4

Greatest to least :

4.75, 4, 3.333..., 2.5

4.75, √16, √10, 10/4

Problem 13 :

Use <, > or = compare.

-√104  ____ -10

Solution :

-√104 = -10...

-√104  < -10

Problem 14 :

Several shops are having sales price are reduced by 62.5%, 2/3, 75%, 1/2 and 7/10. Which list shows the price reductions from greatest to least ?

a)  75%, 62.5%, 1/2, 2/3, 7/10

b)  75%, 7/10, 2/3, 62.5%, 1/2

c)  75%, 7/10, 62.5%, 1/2, 2/3

d)  75%, 7/10, 62.5%, 2/3, 1/2

Solution :

Given prices are,

62.5%, 2/3, 75%, 1/2 and 7/10

62.5% = 62.5/100 = 0.625

2/3 = 0.6666.....

75% = 75/100 = 0.75

1/2 = 0.5

7/10 = 0.7

The order from greatest to least :

0.75, 0.7, 0.6666...., 0.625, 0.5

75%, 7/10, 2/3, 62.5%, 1/2

Problem 15 :

Which is greater ?

a) √0.25    b) √1/4       c) 0.233......

Solution : 

Option c :

Let x = 0.2333....  -----(1)

Multiplying by 10 on both sides.

10x = 2.3333......  -----(2)

(2) - (1)

10x - x = 2.333.... - 0.2333.....

9x = 2.1

x = 2.1/9

x = 21/9

x = 7/3

x = 2.33

2.33 is greater. option c is correct.