COMPARE FRACTIONS WITH DIFFERENT DENOMINATORS

Compare the fractions. Write <,>, or =.

Problem 1:

Solution :

In the fractions 3/4 and 3/8, the denominators are different.

LCM of (4, 8) = 8

3/4 = (3 ∙ 2) / (4 ∙ 2) ==> 6/8

3/8 = (3 ∙ 1) / (8 ∙ 1) = 3/8

Compare the numerators of like fractions 6/8 and 3/8.

6/8 > 3/8

Substitute the corresponding original fractions.

3/4 > 3/8

Problem 2 :

Solution :

In the fractions 2/3 and 4/5, the denominators are different.

LCM of (3, 5) = 15

2/3 = (2 ∙ 5) / (3 ∙ 5) = 10/15

4/5 = (4 ∙ 3) / (5 ∙ 3) = 12/15

Compare the numerators of like fractions 10/15 and 12/15.

10/15 < 12/15

Substitute the corresponding original fractions.

2/3 < 4/5

Problem 3 :

Solution :

In the fractions 1/5 and 2/10, the denominators are different.

LCM of (5, 10) = 10

1/5 = (1 ∙ 2) / (5 ∙ 2) = 2/10

2/10 = (2 ∙ 1) / (10 ∙ 1) = 2/10

Compare the numerators of like fractions 2/10 and 2/10.

2/10 = 2/10

Substitute the corresponding original fractions.

1/5 = 2/10

Problem 4 :

Solution :     

In the fractions 2/10 and 23/100, the denominators are different.

LCM of (10, 100) = 100

2/10 = (2 ∙ 10) / (10 ∙ 10) = 20/100

23/100 = (23 ∙ 1) / (100 ∙ 1) = 23/100

Compare the numerators of like fractions 20/100 and 23/100.

20/100 < 23/100

Substitute the corresponding original fractions.

2/10 < 23/100

Problem 5 :

Solution :

In the fractions 7/8 and 3/4, the denominators are different.

LCM of (8, 4) = 8

7/8 = (7 ∙ 1) / (8 ∙ 1) = 7/8

3/4 = (3 ∙ 2) / (4 ∙ 2) = 6/8

Compare the numerators of like fractions 7/8 and 6/8.

7/8 > 6/8

Substitute the corresponding original fractions.

7/8 > 3/4

Problem 6:

Solution :

In the fractions 7/12 and 5/6, the denominators are different.

LCM of (12, 6) = 12

7/12 = (7 ∙ 1) / (12 ∙ 1) = 7/12

5/6 = (5 ∙ 2) / (6 ∙ 2) = 10/12

Compare the numerators of like fractions 7/12 and 10/12.

7/12 < 10/12

Substitute the corresponding original fractions.

7/12 < 5/6

Problem 7 :

Solution :

In the fractions 10/12 and 5/6, the denominators are different.

LCM of (12, 6) = 12

10/12 = (10 ∙ 1) / (12 ∙ 1) = 10/12

5/6 = (5 ∙ 2) / (6 ∙ 2) = 10/12

Compare the numerators of like fractions 10/12 and 10/12.

10/12 = 10/12

Substitute the corresponding original fractions.

10/12 = 5/6

Problem 8 :

Solution :

In the fractions 53/100 and 1/2, the denominators are different.

LCM of (100, 2) = 100

53/100 = (53 ∙ 1) / (100 ∙ 1) = 53/100

1/2 = (1 ∙ 50) / (2 ∙ 50) = 50/100

Compare the numerators of like fractions 53/100 and 50/100.

53/100 > 50/100

Substitute the corresponding original fractions.

53/100 > 1/2

Problem 9 :

Solution :

In the fractions 2/8 and 9/12, the denominators are different.

Make the denominators same using least common multiple.

LCM of (8, 12) = 24

2/8 = (2 ∙ 3) / (8 ∙ 3) = 6/24

9/12 = (9 ∙ 2) / (12 ∙ 2) = 18/24

Compare the numerators of like fractions 6/24 and 18/24.

6/24 < 18/24

Substitute the corresponding original fractions.

2/8 < 9/12

2/8 is less than 9/12

Problem 10 :

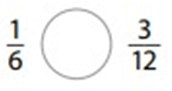
Solution:     

In the fractions 1/6 and 3/12, the denominators are different.

LCM of (6, 12) = 12

1/6 = (1 ∙ 2) / (6 ∙ 2) = 2/12

3/12 = (3 ∙ 1) / (12 ∙ 1) = 3/12

Compare the numerators of like fractions 2/12 and 3/12.

2/12 < 3/12

Substitute the corresponding original fractions.

1/6 < 3/12

Problem 11 :

Solution :

In the fractions 4/5 and 77/100, the denominators are different.

LCM of (5, 100) = 100

4/5 = (4 ∙ 20) / (5 ∙ 20) = 80/100

77/100 = (77 ∙ 1) / (100 ∙ 1) = 77/100

Compare the numerators of like fractions 80/100 and 77/100.

80/100 > 77/100

Substitute the corresponding original fractions.

4/5 > 77/100

4/5 is greater than 77/100

Problem 12:

Solution :     

In the fractions 1/3 and 5/12, the denominators are different.

LCM of (3, 12) = 12

1/3 = (1 ∙ 4) / (3 ∙ 4) = 4/12

5/12 = (5 ∙ 1) / (12 ∙ 1) = 5/12

Compare the numerators of like fractions 4/12 and 5/12.

4/12 < 5/12

Substitute the corresponding original fractions.

1/3 < 5/12