CHECKING IF THE TABLE REPRESENTS DIRECT AND INVERSE VARIATION

Direct variation :

Two variables x and y show direct variation when

y = ax

for some nonzero constant a.

Another type of variation is called inverse variation.

Inverse variation :

Two variables x and y show inverse variation when they are related as follows:

y = a/x, a ≠ 0

The constant a is the constant of variation, and y is said to vary inversely with x.

To check if the table represents direct or inverse variation, we have to consider the following.

Tell whether x and y show direct variation, inverse variation, or neither.

Problem 1 :

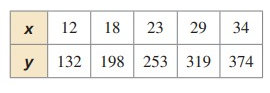
Solution:

Finding the product of x and y :

The product of x and y is not a constant.

Finding the ratio of y to x :

The ratio between y to x is constant. So, the table represents inverse variation.

Problem 2 :

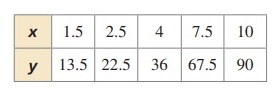
Solution:

Finding the product of x and y :

The product of x and y is not a constant.

Finding the ratio of y to x :

The ratio between y to x is constant. So, the table represents inverse variation.

Problem 3 :

Solution:

Finding the product of x and y :

The product of x and y is constant.

Finding the ratio between y to x :

The ratio between y to x is not constant. So, it is direct variation.

Problem 4 :

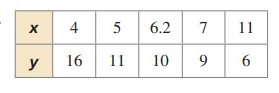
Solution:

Finding the product of x and y :

The product of x and y is not constant.

Finding the ratio of y t ox :

The ratio of y to x is not constant. So, the table represents the neither relationship.