CENTER FOCUS AND DIRECTRIX OF ELLIPSE AND GRAPH WORKSHEET

Graph the ellipse and identify the center, vertices and foci.

Problem 1 :

x29+y225=1

Solution

Problem 2 :

4x2 + 9y2 = 36

Solution

Problem 3 :

x2 + 4y2 = 36

Solution

Problem 4 :

(x+1)2+(y-3)24=1

Solution

Problem 5 :

(x-1)24+(y+2)29=1

Solution

Problem 6 :

36(x + 4)2 + (y + 3)2 = 36

Solution

Answer Key

1)  Ellipse is symmetric about y-axis.

a = 5 and b = 3 

ellipse-q1

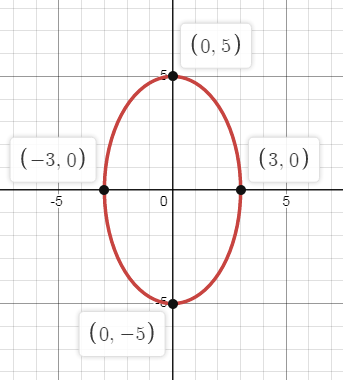
Center: (0, 0)

Vertices: A(0, 5) and A'(0, -5)

Foci: F1(0, 4) F2(0, -4)

2) 

x29+y24=1
ellipse-q2.png

the ellipse symmetric about x-axis.

a = 3 and b = 2

Center: (0, 0)

Vertices: A(3, 0) and A'(-3, 0)

Foci: F1(√5, 0) F2(-√5, 0)

3) 

the ellipse symmetric about x-axis.

a = 6 and b = 3

ellipse-q3.png

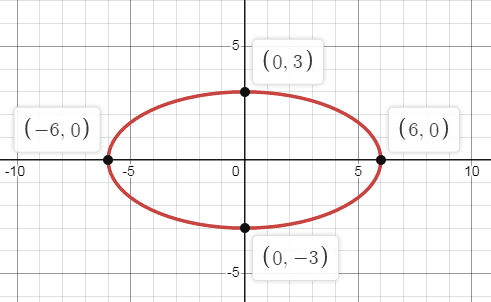
The ellipse symmetric about x-axis.

Center: (0, 0)

Vertices: A (6, 0) and A' (-6, 0)

Foci: F1 (3√3, 0) F2 (-3√3, 0)

4)  

ellipse-q4.png

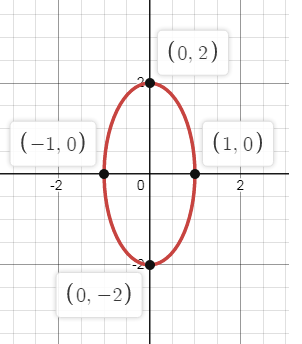
the ellipse symmetric about y-axis.

a = 2 and b = 1

Center: (-1, 3).

Vertices: (-1, 5) and (-1, 1)

Foci: (-1, 3 ± √3)

5)

ellipse-q5.png

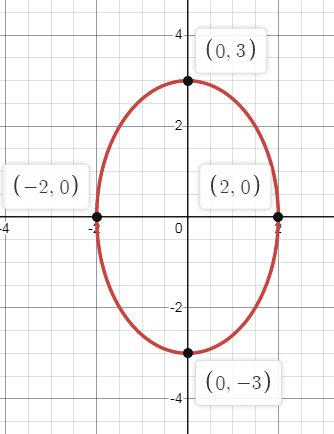
a = 3 and b = 2

Center: (1, -2).

Vertices: (1, 1) and (1, -5).

Foci: (1, -2 ± √5)

6)

ellipse-q6.png

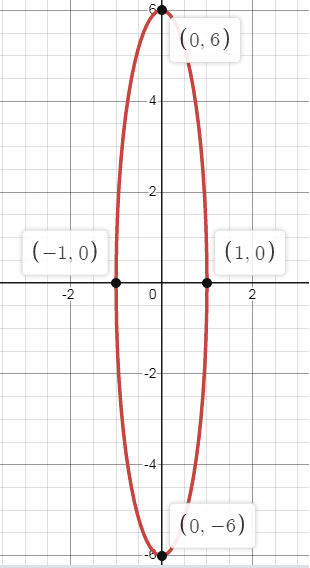
The ellipse symmetric about y-axis.

a = 6 and b = 1

Center: (-4, -3).

Vertices:  (-4, 3) and (-4, -9).

Foci: (-4, -3 ± √35)

Recent Articles

RSS

  1. Finding Range of Values Inequality Problems

    May 21, 24 08:51 PM

    Finding Range of Values Inequality Problems

    Read More

  2. Solving Two Step Inequality Word Problems

    May 21, 24 08:51 AM

    Solving Two Step Inequality Word Problems

    Read More

  3. Exponential Function Context and Data Modeling

    May 20, 24 10:45 PM

    Exponential Function Context and Data Modeling

    Read More