APPLICATION PROBLEMS ON DOT PRODUCT

is the displacement vector of a particle moved from a point to another point after applying a constant force

on the particle, then the work done by the force on the particle is

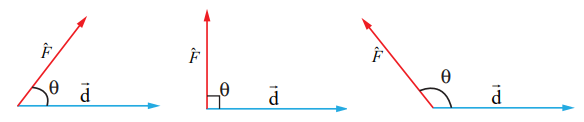
If the force has an acute angle, perpendicular angle, and an obtuse angle, the work done by the force is positive, zero, and negative respectively.

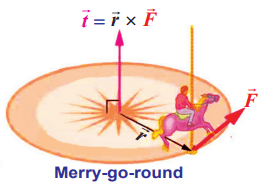
If a force

is applied on a particle at a point with position vector

then the torque or moment on the particle is given by

The torque is called rotational force.

Problem 1 :

Forces of magnitudes 5√2 and 10√2 units acting in the directions

respectively act on a particle which is displaced from the point with position vector

to the point with position vector

Find the work done by the forces.

Solution :

Adding forces :

Difference between position vectors :

Work done :

Problem 2 :

Find the magnitude of the direction cosines of torque represented by

about the position vector

acting through the position vector is

Solution :

Finding magnitude :

Finding Direction Cosine :

Problem 3 :

Find the torque of the resultant of the three forces represented by

acting at the point with position vector

about the point with position vector

Solution :

Finding torque :

Recent Articles