APPLICATION OF DERIVATIVES PROBLEMS ON AP CALCULUS
Problem 1 :
Given f(x) = 2x2 - 7x - 10, find the absolute maximum of f(x) on [-1, 3].
A) -1 B) 7/4 C) -129/8 D) 0
Solution :
f(x) = 2x2 - 7x - 10
f'(x) = 2(2x) - 7(1) - 0
= 4x - 7
= 0
4x - 7 = 0
4x = 7
x = 7/4
At x = -1, f(-1) = 2(-1)2 - 7(-1) - 10
= 2+7-10
= -1
(-1, -1)
At x = 7/4, f(7/4) = 2(7/4)2 - 7(7/4) - 10
= 49/8 - 49/4 - 10
= (49 - 98 - 80)/8
= -129/8
(7/4, -129/8)
At x = 3, f(3) = 2(3)2 - 7(3) - 10
= 18 - 21 - 10
= -13
(3, -13)
So, (-1, -1) is maximum. Option A.
Problem 2 :
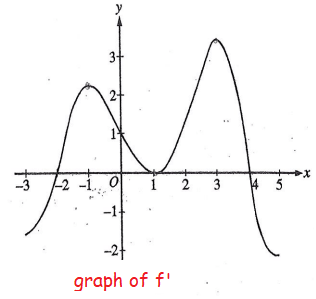
The graph of the derivative of a function f is shown in the figure above. The graph has horizontal tangent lines at x = -1, x = 1 and x = 3. At which of the following values of x does f have relative maximum ?
A) -2 only B) 1 only C) 4 only D) -1 and 3 only
E) -2, 1 and 4.
Solution :
From the graph f'(x), x-intercepts of derivative functions are critical numbers of the original function f(x).
Analyzing the graph of f'(x) :
- Before -2, the curve is below the x-axis. So, in the interval (-∞, -2) f(x) will be decreasing then f(x) will have negative slope.
- After -2 upto 4, the curve is above the x-axis. So, in the interval (-2, 4) f(x) will be increasing then f(x) will have positive slope.
- After 4, the curve is below the x-axis. So, in the interval (4, ∞) f(x) will be decreasing then f(x) will have negative slope.
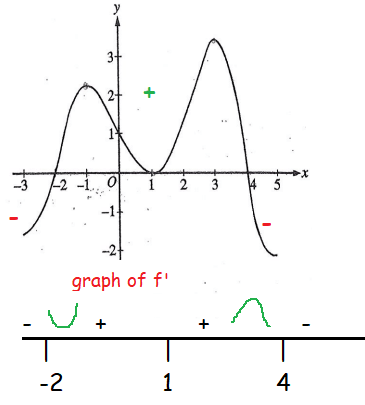
So, maximum value is at x = 4. Option C is correct.
Problem 3 :
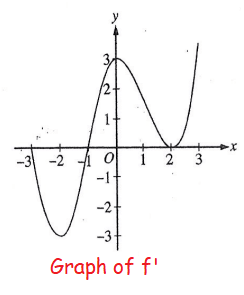
The graph of f', the derivative of the function f is shown above for -3 ≤ x ≤ 3. On what intervals is f increasing ?
A) [-3, -1] only B) [-1, 3] C) [-2, 0] and [2, 3]
D) [-3, -1] and [1, 3]
Solution :
x-intercepts of f'(x) = critical numbers of f(x)
Critical numbers of f(x) :
-3, -1 and 2
Analyzing the graph of f'(x) :
- In between -3 to -1, the curve is below the x-axis. So, in the interval (-3, -1) the function f(x) will be decreasing and f(x) will have negative slope.
- In between -1 and 3, the the curve is above the x-axis. So, in the interval (-1, 3) the function f(x) will be increasing and f(x) will have positive slope.
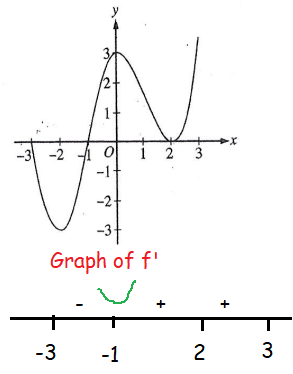
In the interval [-1, 3], the function is increasing.
Problem 4 :
The function f is given by f(x) = x4 + 4x3. On which of the following intervals f is decreasing ?
A) (-3, 0) B) (0, ∞) C) (-3, ∞) D) (-∞, -3)
Solution :
f(x) = x4 + 4x3
f'(x) = 4x3 + 4(3x2)
= 4x3 + 12x2
f'(x) = 0
4x2 (x + 3) = 0
x = 0 and x = -3
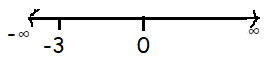
(-∞, -3) (-3, 0) and (0, ∞)
|
-4 ∈ (-∞, -3) |
f'(x) = 4x2 (x + 3) x = -4 f'(-4) = 4(-4)2 (-4 + 3) - < 0 Decreasing on (-∞, -3) |
|
-2 ∈ (-3, 0) |
f'(x) = 4x2 (x + 3) x = -2 f'(-2) = 4(-2)2 (-2 + 3) + > 0 Increasing on (-3, 0) |
|
1 ∈ (0, ∞) |
f'(x) = 4x2 (x + 3) x = 1 f'(1) = 4(1)2 (1 + 3) + > 0 Increasing on (0, ∞) |
Decreasing on the interval (-∞, -3). Option A is correct.
Problem 5 :
Let f be the function with derivative given by
On what intervals is f decreasing ?
A) (0, ∞) only B) (-∞, 0] only C) [-1/√3, 1/√3] only
D) (-∞, ∞) E) There is no such interval
Solution :
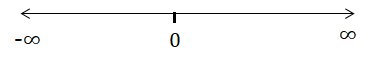
|
-2 ∈ (-∞, 0) |
f'(x) = + > 0 Increasing on (-∞, 0) |
|
1 ∈ (0, ∞) |
f'(x) = - < 0 Decreasing on (0, ∞) |
Decreasing on (0, ∞) only. Option A is correct.
Problem 6 :
Identify the open intervals where the function
f(x) = x√(30 - x2)
is increasing or decreasing.
A) Decreasing (-∞, √15); increasing (√15, ∞)
B) Decreasing (-∞, ∞)
C) Decreasing (√30, ∞); increasing (-∞, √30)
D) Decreasing (-√30, -√15) U (√15, √30); increasing (-√15, √15)
E) Decreasing (-√15, √15); increasing (-√30, -√15) U (√15, √30) (-√15, √15)
Solution :
f(x) = x√(30 - x2)
Increasing or decreasing intervals :
So, option D
Decreasing (-√30, -√15) U (√15, √30); increasing (-√15, √15)
is correct.
Recent Articles
-
Finding Range of Values Inequality Problems
May 21, 24 08:51 PM
Finding Range of Values Inequality Problems -
Solving Two Step Inequality Word Problems
May 21, 24 08:51 AM
Solving Two Step Inequality Word Problems -
Exponential Function Context and Data Modeling
May 20, 24 10:45 PM
Exponential Function Context and Data Modeling