SAT PRACTICE QUESTIONS ON SHAPE

Problem 1 :

What is the radius of a circle that has a circumference of π?

A) 1/4      B) 1/2     C) 1     D) 2     E) 4

Solution:

Given that the circumference of circle is π.

We know that the circumference of circle is 2πr, where r is radius of circle.

 Circumference = π = 2πr

r = 1/2

So, option (B) is correct.

Problem 2 :

In the figure above, arc SBT is one quarter of a circle with center R and radius 6. If the length plus the width of rectangle ABCR is 8, then the perimeter of the shaded region is

A) 8 + 3π        B) 10 + 3π      C) 14 + 3π     D) 1 + 6π

E) 12 + 6π

Solution:

Given, radius = 6

AS = 6 - a , CT = 6 - b , AC = RB = 6 ( radius )

Perimeter of the shaded region = AS + AC + CT + C(SBT)

Perimeter = ( 6 - a ) + 6 + ( 6 - b ) + 3π

Perimeter = 18 - ( a + b ) + 3π

length + width = 8

Perimeter = 18 - 8 + 3π

 Perimeter = 10 + 3π

So, option (B) is correct.

Problem 3 :

In the xy-coordinate plane, what is the area of the square with opposite vertices at (-2, -2) and (2, 2) ?

A) 4    B) 8    C) 16     D) 32   E) 64

Solution:

By finding the distance between the above two points, we get the side length of the square.

So, option (D) is correct.

Problem 4 :

In rectangle ABCD, point E is the midpoint of BC. If the area of quadrilateral ABED is 2/3, what is the area of rectangle ABCD?

A)  1/2      B)  3/4      C)  8/9     D)  1       E)  8/3

Solution :

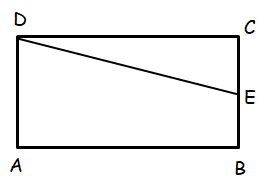
Area of rectangle = AB x AD

Area of quadrilateral = 1/2 x height x sum of parallel sides

1/2 x AB x (AD + BE) = 2/3

1/2 x AB x (AD + 1/2 x AD) = 2/3

1/2 x AB x (3/2 x AD) = 2/3

AB x AD = (2/3) x (4/3)

AB x AD = (8/9)

So, option C is correct.

Problem 5 :

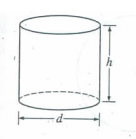
In right circular cylinder above has diameter d and height h. Of the following expressions, which represents the volume of the smallest rectangular box that completely contains the cylinder?

A) dh      B) d²h       C) dh²      D) d²h²       E) (d + h)²

Solution:

Diameter of the cylinder will be the width of the rectangular box, length of the rectangular box is h.

Volume of rectangular box = length x width x height

= h x d x d

= h d²

So, option B is correct.

Problem 6 :

If the volume of a cube is 8, what is the shortest distance from the center of the cube to the base of the cube?

A) 1     B) 2    C) 4    D) √2    E) 2√2   

Solution:

Given, volume of a cube = 8

Volume of cube = a³

a³ = 8

a = 2

Shortest distance from the center of the cube to the base is half of its side length a

= a/2

= 2/2

= 1 unit

So, option (A) is correct.

Problem 7 :

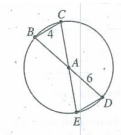
In the figure above, point A is the center of the circle and segments BD and CE are diameters. Which of the following statements is true?

A) CA > 6    B) ED > 4    C) BA  < 4   D) CA = 4   E) ED = 4

Solution:

In triangles AED and BCA.

AD = AE = AC = AB (radii)

∠EAD = ∠BAC (A)

AE = AC (S)

AD = AB (S)

Using SAS, the triangles are congruent.

So, ED = 4, option E.

Problem 8 :

In the figure above, the two circles are tangent at point B and AC = 6. If the circumference of the circle with center A is twice the circumference of the circle with center C, what is the length of BC?

A) 1    B) 2    C) 3     D) 4    E) 6

Solution:

Let BC (r) be the radius of smaller circle and AB (R) is the radius of the larger circle.

R + r = 6

Circumference of the larger circle = 2(circumference of the smaller circle)

2πR = 2(2πr)

R = 2r

R = 2(6-R)

R = 12 - 2R

3R = 12

R = 4

BC = r 

AB + BC = 6

R + r = 6

BC = 6 - 4

BC = 2

Problem 9 :

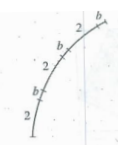
The figure above shows part of a circle whose circumference is 45. If arcs of length 2 and length b continue to alternate around the entire circle so that there are 18 arcs of each length, what is the degree measure of each of the arcs of length b ?

A) 4°    B) 6°    C) 10°    D) 16°    E) 20°

Solution:

You know that 18(2 + b) = 45, so you can calculate b:

18(2 + b) = 45

36 + 18b = 45

18b = 9

b = 0.5

0.5/45 = x/360

x = 4

Problem 10 :

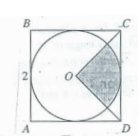
In the figure above, the circle with center O is inscribed in square ABCD. What is the area of the shaded portion of the circle?

Solution:

Area of sector = θ/360  πr²

Side length of the square = diameter of the circle

radius = 1

= (90/360) π1(1)²

= π/4