7TH GRADE STAAR MATH PRACTICE

Problem 21 :

A sidewalk in the shape of two triangles, a rectangle, and a square was built around the edge of a building as shown.

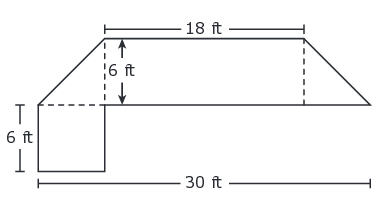
What is the area of the sidewalk in square feet?

A. 108 ft²      B. 162 ft²         C. 144 ft²     D. 180 ft²

Solution

Problem 22 :

The price of a computer is $899.00. The sales tax rate is 7%. What is the sales tax on this computer in dollars and cents?

Solution

Problem 23 :

An online game increases in number of users at a rate of 500 users each day. Which graph best represents the relationship between y, the number of users and x, the number of days?

Solution

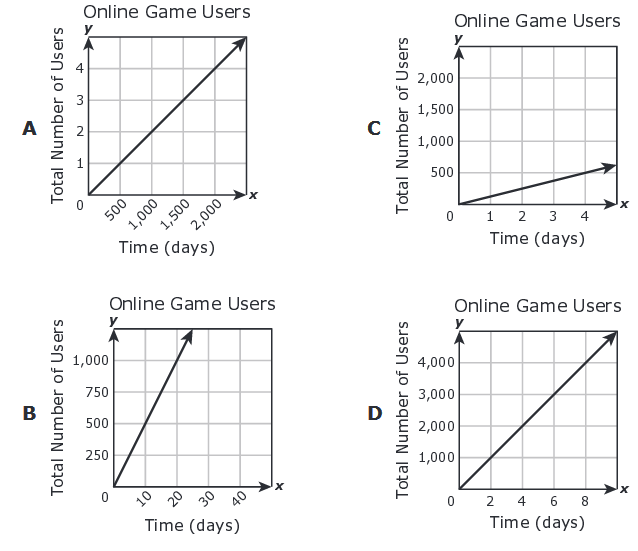
Problem 24 :

The radius of circle S is half the radius of circle L. The radius of circle L is 8 millimeters.

Which measurement is closest to the area of circle S in square millimeters?

A. 50.24 mm²     B. 25.12 mm²      C. 200.96 mm²   

D.12.56 mm²

Problem 25 :

Which situation is best represented by the following equation?

68.50x + 127.95 = 675.95

A. An office manager paid $675.95 to build a web site. The office manager bought a software package for $68.50 and paid an employee $127.95 for each hour she worked on the website. What is x, the number of hours the employee worked on the website?

B. An office manager paid $675.95 for computer equipment. The office manager bought one monitor for $127.95 and hard drives for $68.50 each. What is x, the number of hard drives the office manager bought?

C. A sales manager paid $675.95 for advertising. The sales manager paid $127.95 per hour for consulting and received a $68.50 discount. What is x, the number of hours the manager paid for consulting?

D. A business owner paid a total of $675.95 for two employees to work the same number of days. The business owner paid one employee $68.50. The business paid a second employee $127.95 per day. What is x, the number of days the employees worked?

Solution

Problem 26 :

Regina has three number cubes. The faces of each number cube are numbered from 1 to 6. Regina will roll each number cube one time.

What is the probability that all three number cubes will land on an odd number?

Solution

Problem 27 :

What is the solution set for this inequality?

Solution

Problem 28 :

The net of a triangular prism and its approximate dimensions are shown in the diagram.

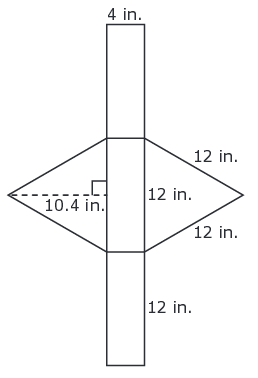
Which measurement is closest to the total surface area of the triangular prism in square inches?

A. 268.8 in²      B. 432 in²    C. 288 in²   D. 393.6 in²

Problem 29 :

The manager of a coffee shop recorded the number of customers who put vanilla creamer or chocolate creamer in their coffee during one hour and classified them by age. The results are shown in the table.

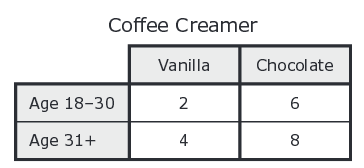
What percentage of these customers put chocolate creamer in their coffee during this hour?

A. 30%     B. 14%    C. 70%    D. 75%

Solution

Problem 30 :

An engineer created a scale drawing of a building using a scale in which 0.25 inch represents 2 feet. The length of the actual building is 250 feet.

What is the length in inches of the building in the scale drawing?

Solution

Problem 31 :

The box plots summarize the number of semester hours students enrolled in a university and a community college completed during the fall semester.

Which statement is best supported by the data in the box plots?

A. The median of the data for the university is greater than the median of the data for the community college.

B. The range of the data for the university is greater than the range of the data for the community college.

C. The interquartile range of the data for the community college is greater than the interquartile range of the data for the university.

D. The third quartile of the data for the community college is greater than the third quartile of the data for the university

Solution

Problem 32 :

Alice has a loan of $24,820. This loan has a simple interest rate of 3.5% per year. No payments will be made on the loan until the end of one year.

How much interest will Alice pay on this loan at the end of one year?

A. $868.70     B. $72.39    C. $8,687.00   D. $25,688.70

Solution

Problem 33 :

A student has a set of cards. Each card has a picture of one shape. The table shows the number of cards that have a picture of each shape. The student will randomly select one card from the set.

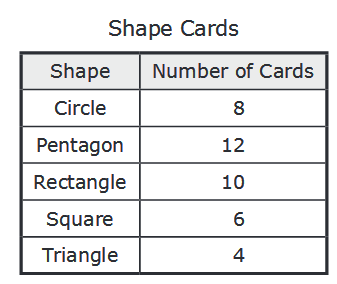
Which statement is true?

A. The probability of selecting a card with a picture of a circle is 5/8, and the probability of selecting a card that is not a picture of a circle is 3/8.

B. The probability of selecting a card with a picture of a circle is 3/8, and the probability of selecting a card that is not a picture of a circle is 5/8.

C. The probability of selecting a card with a picture of a circle is 1/5, and the probability of selecting a card that is not a picture of a circle is 4/5.

D. The probability of selecting a card with a picture of a circle is 4/5, and the probability of selecting a card that is not a picture of a circle is 1/5.

Solution

Problem 34 :

The model represents an equation.

What is the solution for the equation?

Solution

Problem 35 :

A survey showed that 8 out of 20 homeowners in a neighborhood had cable television. If there were 320 homeowners in the neighborhood, how many could be expected to have cable television?

Solution

Problem 36 :

Students were surveyed to determine their favorite types of animals. The bar graph shows the number of students who selected each type of animal

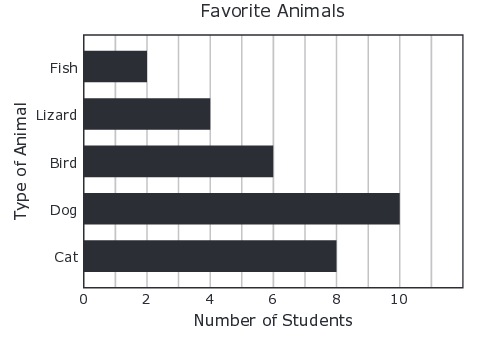
What percentage of the students surveyed selected “Bird” as their favorite type of animal?

A. 20%    B. 5%      C. 6%     D. 80%

Solution

Problem 37 :

A principal has given a class $75 to help pay for a field trip to a zoo. The students in the class are selling pies for $5 each to earn the rest of the money they need. The field trip will cost a total of $386.

Which inequality can be used to find p, the number of pies the class needs to sell in order to earn enough money to pay for the field trip?

A. 5p + 75 ≤ 386         B. 5p + 75 ≥ 386

C. 75p + 5 ≥ 386         D. 75p + 5 ≤ 386

Solution

Problem 38 :

The dimensions of a rectangular prism are 1.5 feet by 3.5 feet by 2 feet. What is the volume of the rectangular prism in cubic feet?

A. 7 ft³    B. 7.25 ft³          C. 8.5 ft³           D. 10.5 ft³

Solution

Problem 39 :

The circumference of a circle is C inches. The diameter of the circle is 19 inches. Which expression best represents the value of π?

Solution

Problem 40 :

A monthly budget for a small family is shown.

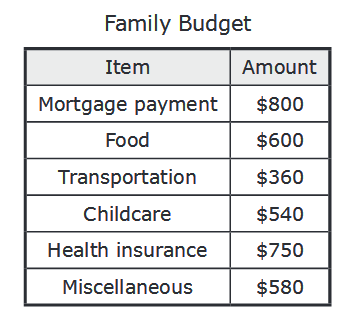
Which equation can be used to find b, the minimum amount of money the family must earn annually in order to meet this budget?

A. b = $3,630 × 12              B. b = $3,630 × 52

C. b = $43,560 ÷ 52           D. b = $43,560 ÷ 365

Solution